inellipses are the Steiner inellipse, which touches the triangle at the midpoints of its sides, the Mandart inellipse and Brocard inellipse (see examples section)...

In geometry, the Steiner inellipse, midpoint inellipse, or midpoint ellipse of a triangle is the unique ellipse inscribed in the triangle and tangent...

vertices z1, z2, z3 and tangent to the sides at their midpoints: the Steiner inellipse. The foci of that ellipse are the zeroes of the derivative p'(z). This...

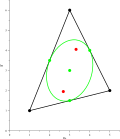
In geometry, the Mandart inellipse of a triangle is an ellipse that is inscribed within the triangle, tangent to its sides at the contact points of its...

triangle and tangent to all three sides. Every triangle has a unique Steiner inellipse which is interior to the triangle and tangent at the midpoints of the...

{\displaystyle c} remains constant. Every triangle has a unique Steiner inellipse – an ellipse inside the triangle and tangent to the midpoints of the three...

called the Steiner circumellipse, to distinguish it from the Steiner inellipse. Named after Jakob Steiner, it is an example of a circumconic. By comparison...

triangle, the inellipse with its center at that point is unique.: p.142  The inellipse with the largest area is the Steiner inellipse, also called the...

(non-Euclidean geometry) Hypotenuse Incircle and excircles of a triangle Inellipse Integer triangle Isodynamic point Isogonal conjugate Isoperimetric point...

vertices at the feet of the altitudes), and the only triangle whose Steiner inellipse is a circle (specifically, the incircle). The triangle of the largest...

the roots of the derivative of the cubic are the foci of the Steiner inellipse of the triangle—the unique ellipse that is tangent to the triangle at...

\triangle T_{A}T_{B}T_{C}} is called the Mandart circle (cf. Mandart inellipse). The three line segments A T A ¯ {\displaystyle {\overline {AT_{A}}}}...

the centroid, inellipses: ellipses which touch the sides of a triangle. Special cases are the Steiner inellipse and the Mandart inellipse. Ellipses appear...

that are dual in this way include the Steiner ellipse and the Steiner inellipse, and the Kiepert hyperbola and the Kiepert parabola. In the following...

the Gergonne point. The mittenpunkt is also the centroid of the Mandart inellipse of the given triangle, the ellipse tangent to the triangle at its extouch...

neighbored tangents coincide. This procedure results in a statement on inellipses of triangles. From a projective point of view the two triangles P 1 P...

triangle. Every triangle has an inscribed ellipse, called its Steiner inellipse, that is internally tangent to the triangle at the midpoints of all its...

inscribed ellipses. One of them is a circle, and one of them is the Steiner inellipse which is tangent to the triangle at the midpoints of the sides. Every...

the triangle with these points as its vertices has a unique Steiner inellipse that is tangent to the triangle's sides at their midpoints. The major...

vertex of the extouch triangle and one of the points where the Mandart inellipse is tangent to the triangle side. The three splitters concur at the Nagel...

S2CID 12307207. Scimemi, Benedetto, "Simple Relations Regarding the Steiner Inellipse of a Triangle", Forum Geometricorum 10, 2010: 55–77. An interactive applet...

orthic inconic that is tangent to the sides of the triangle △ABC is an inellipse and the orthic inconics of the other three possible triangles are hyperbolas...

Poncelet–Steiner theorem Parallel axes rule Steiner–Lehmus theorem Steiner inellipse Steinerian Steiner point (computational geometry) Steiner point (triangle)...

Marden's theorem states that the zeros of P' are the foci of the Steiner inellipse which is the unique ellipse tangent to the midpoints of the triangle formed...

also made by August Leopold Crelle and Carl Gustav Jacob Jacobi. Mandart inellipse Trisected perimeter point Dussau, Xavier (April 2020). "Elementary construction...

excircles of a triangle, as well as the triangle's inconics that are not inellipses, are externally tangent to one side and to the other two extended sides...

point. This is shown in blue and labelled "N" in the diagram. The Mandart inellipse is tangent to the sides of the reference triangle at the three vertices...

233, Lemma 1  A point in the interior of a triangle is the center of an inellipse of the triangle if and only if the point lies in the interior of the medial...

Banach–Mazur compactum – Concept in functional analysis Ellipsoid method Steiner inellipse, the special case of the inner Löwner–John ellipsoid for a triangle. Fat...