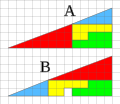
In geometry, Pick's theorem provides a formula for the area of a simple polygon with integer vertex coordinates, in terms of the number of integer points...
20 KB (2,339 words) - 01:48, 17 December 2024
Schleisinger and Adolf Josef Pick and died at Theresienstadt concentration camp. Today he is best known for Pick's theorem for determining the area of...
4 KB (339 words) - 10:13, 4 June 2025
Area of a triangle (section Using Pick's theorem)
side lengths (Heron's formula), vectors, coordinates, line integrals, Pick's theorem, or other properties. Heron of Alexandria found what is known as Heron's...
21 KB (3,572 words) - 04:47, 6 June 2025
Schwarz lemma (redirect from Schwarz-Pick theorem)
desired. A variant of the Schwarz lemma, known as the Schwarz–Pick theorem (after Georg Pick), characterizes the analytic automorphisms of the unit disc...
9 KB (1,728 words) - 21:29, 22 June 2025
Integer lattice (section Pick's theorem)
lattice is coarsely equivalent to Euclidean space. Pick's theorem, first described by Georg Alexander Pick in 1899, provides a formula for the area of a simple...
5 KB (516 words) - 09:52, 5 April 2024
Schwarz–Ahlfors–Pick theorem is an extension of the Schwarz lemma for hyperbolic geometry, such as the Poincaré half-plane model. The Schwarz–Pick lemma states...
2 KB (214 words) - 00:33, 12 August 2023
in 1957 used them to show that higher-dimensional generalizations of Pick's theorem do not exist. Despite this negative result, Reeve developed an alternative...
7 KB (880 words) - 00:14, 27 May 2025
Ehrhart polynomial (section The Betke–Kneser theorem)
Ehrhart polynomials can be seen as a higher-dimensional generalization of Pick's theorem in the Euclidean plane. These polynomials are named after Eugène Ehrhart...
16 KB (2,245 words) - 15:01, 9 July 2025
has motivated various attempts to generalise Nevanlinna and Pick's result. The Nevanlinna–Pick problem can be generalised to that of finding a holomorphic...
6 KB (1,157 words) - 00:21, 15 April 2025
by Alicia K. Harris Pick operating system, a computer operating system Pick's disease, a neurodegenerative disease Pick's theorem in geometry Sertoli...
3 KB (384 words) - 04:48, 17 May 2025
fairy chess piece the wazir form a square lattice graph. Lattice path Pick's theorem Integer triangles in a 2D lattice Regular graph Weisstein, Eric W. "Lattice...
4 KB (547 words) - 11:50, 28 June 2025
Minkowski's second theorem (geometry of numbers) Minkowski–Hlawka theorem (geometry of numbers) Monsky's theorem (discrete geometry) Pick's theorem (geometry)...
78 KB (6,296 words) - 20:31, 6 July 2025
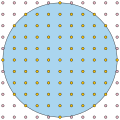
red dots in the above image) with an area of exactly one grid square (Pick's theorem gives 0 + 4/2 − 1 = 1), which corresponds to the "missing" area. According...
8 KB (835 words) - 21:59, 24 June 2025
digital images). Study of properties of digital sets; see, for example, Pick's theorem, digital convexity, digital straightness, or digital planarity. Transforming...
8 KB (980 words) - 17:06, 29 July 2023
conjectured to be PPP complete. Danzer set Pick's theorem Dirichlet's unit theorem Minkowski's second theorem Ehrhart's volume conjecture Olds, C. D.; Lax...
19 KB (2,350 words) - 11:23, 30 June 2025
angle Holditch's theorem Interactive geometry software Involutes Goat grazing problem Parallel postulate Polygon Star polygon Pick's theorem Shape dissection...
13 KB (938 words) - 15:07, 19 June 2025
the origin in the square of side 2n, centered at the origin. Using Pick's theorem, the area of the sunburst is 4(|Fn| − 1), where |Fn| is the number of...
41 KB (5,077 words) - 22:13, 8 May 2025
_{F}v_{1}\wedge v_{2}\wedge v_{3}\right\|} Planimeter Polygon area Pick's theorem Heron's formula Mathologer video about Gauss's shoelace formula Bart...
17 KB (3,779 words) - 02:45, 13 May 2025
polygon and b is the number of boundary points. This result is known as Pick's theorem. The area between a positive-valued curve and the horizontal axis, measured...
43 KB (5,365 words) - 07:16, 30 April 2025
polygon. These include the shoelace formula for arbitrary polygons, and Pick's theorem for polygons with integer vertex coordinates. The convex hull of a simple...
31 KB (3,206 words) - 07:42, 13 March 2025
numbers Minkowski's theorem Pick's theorem Mahler's compactness theorem Mahler measure Effective results in number theory Mahler's theorem Brun sieve Function...
10 KB (937 words) - 18:05, 24 June 2025
measurement with random placements. According to Pick's theorem, published by Georg Alexander Pick in 1899, the version of the dot planimeter with boundary...
10 KB (1,040 words) - 01:56, 17 December 2024
an equally spaced grid such that all its vertices are grid points, Pick's theorem gives a simple formula for the polygon's area based on the numbers of...
37 KB (4,236 words) - 14:28, 13 January 2025
the area of a shape by counting the lattice points that it contains Pick's theorem, a more precise relationship between area and lattice points covered...
15 KB (1,935 words) - 00:55, 16 February 2025
geometry: Polyhedral combinatorics Lattice polytopes Ehrhart polynomials Pick's theorem Hirsch conjecture Opaque set Packings, coverings, and tilings are all...
15 KB (1,575 words) - 05:36, 16 October 2024
Ren, Ding; Reay, John R. (1987). "The boundary characteristic and Pick's theorem in the Archimedean planar tilings". J. Comb. Theory A. 44 (1): 110–119...
32 KB (2,009 words) - 01:08, 16 April 2025
In vector calculus, the divergence theorem, also known as Gauss's theorem or Ostrogradsky's theorem, is a theorem relating the flux of a vector field through...
45 KB (7,538 words) - 18:50, 5 July 2025
Poincaré–Hopf theorem (also known as the Poincaré–Hopf index formula, Poincaré–Hopf index theorem, or Hopf index theorem) is an important theorem that is used...
7 KB (926 words) - 22:20, 1 May 2025
polytopes and the Dehn–Sommerville equations relating numbers of faces; Pick's theorem and the Ehrhart polynomials, both of which relate lattice counting to...
4 KB (384 words) - 02:18, 17 September 2024
relation bc − ad = 1 holds. This fact may be deduced e.g. with the help of Pick's theorem which expresses the area of a plane triangle whose vertices have integer...
11 KB (2,040 words) - 14:58, 3 June 2025