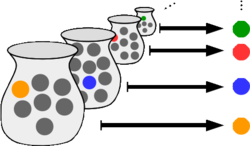
mathematics, an additive set function is a function μ \mu mapping sets to numbers, with the property that its value on a union of two disjoint sets equals the...

of a statistical trial or experiment Sigma-additive set function – Mapping function Sigma-ring – Family of sets closed under countable unions Elstrodt...

be a set and Σ {\displaystyle \Sigma } a σ-algebra over X . {\displaystyle X.} A set function μ {\displaystyle \mu } from Σ {\displaystyle \Sigma } to...

mathematics, especially measure theory, a set function is a function whose domain is a family of subsets of some given set and that (usually) takes its values...

addition operation Additive set-function see Sigma additivity Additive category, a preadditive category with finite biproducts Additive inverse, an arithmetic...

may appear reasonable. However the closest generalization to mass is sigma additivity, which gives rise to the Lebesgue measure. It assigns a measure of...

an atom-free vector-valued sigma-additive set function. Here, a function from a family of sets to vectors is sigma-additive when the family is closed under...

generalized additive model (GAM) is a generalized linear model in which the linear response variable depends linearly on unknown smooth functions of some...

Delta-sigma (ΔΣ; or sigma-delta, ΣΔ) modulation is an oversampling method for encoding signals into low bit depth digital signals at a very high sample-frequency...

{B(\alpha _{t}+i\sigma _{t}u,\beta _{t}-i\sigma u)}{B(\alpha _{t},\beta _{t})}}\right)^{\delta _{t}}e^{i\mu _{t}u}\;\;.} Two subcases of additive logistic process...

In statistics, an additive model (AM) is a nonparametric regression method. It was suggested by Jerome H. Friedman and Werner Stuetzle (1981) and is an...

processing and statistics, a window function (also known as an apodization function or tapering function) is a mathematical function that is zero-valued outside...

particularly norms and square roots. Additive maps are special cases of subadditive functions. A subadditive function is a function f : A → B {\displaystyle f\colon...

is no prime number that divides both of them. Then an arithmetic function a is additive if a(mn) = a(m) + a(n) for all coprime natural numbers m and n;...

{y-\mu }{\sigma }}} . Other definitions of the Q-function, all of which are simple transformations of the normal cumulative distribution function, are also...

not a result. A cylinder set measure can be intuitively understood as defining a finitely additive function on the cylinder sets of the topological vector...

Harish-Chandra's σ function Weierstrass sigma function Sigma additivity Sigma (album) Sigma (DJs), a British drum and bass duo Sigma AZ (band), punk rock...

field of measure theory, τ-additivity is a certain property of measures on topological spaces. A measure or set function μ {\displaystyle \mu } on a...

In mathematics, a nonempty collection of sets is called a 𝜎-ring (pronounced sigma-ring) if it is closed under countable union and relative complementation...

ω ( n ) {\displaystyle \gamma (n)=(-1)^{\omega (n)}} , where the additive function ω ( n ) {\displaystyle \omega (n)} is the number of distinct primes...

cumulative distribution function is F X ( x ) = Φ ( ln ⁡ x − μ σ ) {\displaystyle F_{X}(x)=\Phi {\left({\frac {\ln x-\mu }{\sigma }}\right)}} where Φ {\displaystyle...

In economics, additive utility is a cardinal utility function with the sigma additivity property.: 287–288  Additivity (also called linearity or modularity)...

algebra 𝜎-ring – Family of sets closed under countable unions Sigma additivity – Mapping functionPages displaying short descriptions of redirect targets Bauer...

dual: the additive form. If Y is reproductive, then Z = λ Y {\displaystyle Z=\lambda Y} with λ = 1 σ 2 {\displaystyle \lambda ={\frac {1}{\sigma ^{2}}}}...

E\in \Sigma } Definition 1.3. The variation (also called absolute variation) of the signed measure μ {\displaystyle \mu } is the set function | μ | (...

club set stratified A formula of set theory is stratified if and only if there is a function σ {\displaystyle \sigma } which sends each variable appearing...

{\displaystyle \sigma } -finite), and moreover that it does not fail to satisfy the sigma-additivity of the original function. For a given set Ω , {\displaystyle...

log-likelihood function, i.e. the cost function: Ignoring constants independent of θ {\displaystyle \theta } and σ {\displaystyle \sigma } , Equation (cpd...

{\displaystyle (\forall x^{\sigma })(\exists y^{\tau })R(x,y)\to (\exists f^{\sigma \to \tau })(\forall x^{\sigma })R(x,f(x)).} Unlike in set theory, the axiom...

i_{1}<i_{2}<\cdots <i_{k}}\sigma _{i_{1}}\sigma _{i_{2}}\cdots \sigma _{i_{k}}} This is the k {\textstyle k} th elementary symmetric function of the singular values...