s⋯21. The Erdős–Szekeres theorem can be proved in several different ways; Steele (1995) surveys six different proofs of the Erdős–Szekeres theorem, including...
10 KB (1,174 words) - 15:57, 18 May 2024
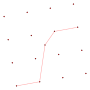
Happy ending problem (redirect from Erdős-Szekeres conjecture)
problem" (so named by Paul Erdős because it led to the marriage of George Szekeres and Esther Klein) is the following statement: Theorem — any set of five points...
18 KB (1,879 words) - 22:55, 14 November 2024
theorem relating longest paths and colorings in graphs, and to the Erdős–Szekeres theorem on monotonic subsequences. The height of a partially ordered set...
8 KB (1,119 words) - 13:33, 10 November 2023
number Szekeres snark Generalized continued fraction Kruskal–Szekeres coordinates Szekeres–Wilf number Schröder's equation Erdős–Szekeres theorem Obituary...
7 KB (636 words) - 04:25, 25 April 2024
Robinson-Schensted correspondence can be used to give a simple proof of the Erdős–Szekeres theorem. Viennot's geometric construction, which provides a diagrammatic...
14 KB (1,795 words) - 06:10, 25 October 2023
theorem Erdős–Szekeres theorem Erdős–Szemerédi theorem Erdős–Tetali theorem Erdős–Wintner theorem Erdős–Turán inequality Erdős–Ulam problem Erdős–Woods number...
3 KB (234 words) - 23:16, 10 August 2024
(combinatorics) Erdős–Nagy theorem (discrete geometry) Erdős–Pósa theorem (graph theory) Erdős–Rado theorem (set theory) Erdős–Stone theorem (graph theory) Erdős–Szekeres...
73 KB (6,038 words) - 09:58, 20 November 2024
theorems in combinatorics, including Dilworth's theorem and Mirsky's theorem on partially ordered sets, Kőnig's theorem on matchings, and the Erdős–Szekeres...
59 KB (7,054 words) - 11:33, 16 December 2024
statement of the theorem to require, in a simple graph, either an r-clique or an s-independent set, see (Gross 2008) or (Erdős & Szekeres 1935). In this...
65 KB (8,171 words) - 17:35, 8 December 2024
the form m2i. Therefore, by Dilworth's theorem, the width of this partial order is n. The Erdős–Szekeres theorem on monotone subsequences can be interpreted...
18 KB (2,429 words) - 21:37, 20 November 2024
Subsequence (section Theorems)
decreasing subsequence of length s {\displaystyle s} (This is the Erdős–Szekeres theorem). A metric space ( X , d ) {\displaystyle (X,d)} is compact if every...
6 KB (828 words) - 21:48, 25 September 2022
mathematician Paul Erdős and his various collaborators made many famous mathematical conjectures, over a wide field of subjects, and in many cases Erdős offered...
14 KB (1,438 words) - 16:40, 31 October 2024
time by dynamic programming for points in convex position. The Erdős–Szekeres theorem guarantees that every set of n {\displaystyle n} points in general...
5 KB (545 words) - 10:14, 18 December 2023
which all slopes have the same sign. This is a corollary of the Erdős–Szekeres theorem. Polygonal chains can often be used to approximate more complex...
9 KB (1,005 words) - 01:57, 21 October 2024
Ramsey theory (redirect from Ramsey-type theorem)
(1): 264–286, doi:10.1112/plms/s2-30.1.264 (behind a paywall). Erdős, Paul; Szekeres, George (1935), "A combinatorial problem in geometry", Compositio...
9 KB (1,143 words) - 05:04, 16 December 2024
O ( n ) {\displaystyle O(n)} term. Example run According to the Erdős–Szekeres theorem, any sequence of n 2 + 1 {\displaystyle n^{2}+1} distinct integers...
20 KB (2,446 words) - 08:50, 7 October 2024
Degeneracy (graph theory) (redirect from Szekeres-Wilf number)
and is essentially the same as the coloring number or Szekeres–Wilf number (named after Szekeres and Wilf (1968)). k-degenerate graphs have also been called...
29 KB (3,395 words) - 01:38, 10 November 2024
suggested a conjecture offering a modular strengthening of the Erdős–Szekeres theorem proving that the number of points in the interior of the polygon...
14 KB (1,185 words) - 05:25, 23 July 2024
Mathematics Paul Erdős and Richard Rado Erdős–Stone theorem Mathematics Paul Erdős and Arthur Harold Stone Erdős–Szekeres theorem Mathematics Paul Erdős and George...
21 KB (100 words) - 23:09, 21 December 2024
at least to the graph-theoretic reformulation of Ramsey theory by Erdős & Szekeres (1935), the term clique comes from Luce & Perry (1949), who used complete...
20 KB (2,495 words) - 09:58, 16 December 2024
761–763, doi:10.2307/2310461, JSTOR 2310461 Szekeres, E.; Szekeres, G. (1965), "On a problem of Schütte and Erdős", The Mathematical Gazette, 49: 290–293...
18 KB (2,546 words) - 13:35, 4 October 2024
University Press, pp. 44–46. Morris, Walter D.; Soltan, Valeriu (2000), "The Erdős-Szekeres problem on points in convex position—a survey", Bull. Amer. Math. Soc...
190 KB (19,551 words) - 10:14, 23 December 2024
This is the first exponential improvement over the upper bound of Erdős and Szekeres, proved in 1935. Sahasrabudhe has also worked with Marcelo Campos...
13 KB (1,319 words) - 18:19, 29 October 2024
early landmark result in the field is the Erdős–Szekeres theorem; in permutation pattern language, the theorem states that for any positive integers a and...
35 KB (4,037 words) - 21:57, 2 November 2024
first professor of applied mathematics George Szekeres – mathematician known for the Erdős–Szekeres theorem Ernie Tuck – applied mathematician Mathai Varghese...
41 KB (3,114 words) - 15:11, 11 December 2024
4321, 1234 1, 2, 6, 22, 86, 306, 882, 1764, ... A206736 finite Erdős–Szekeres theorem 4312, 1234 1, 2, 6, 22, 86, 321, 1085, 3266, ... A116705 polynomial...
29 KB (1,372 words) - 21:57, 2 November 2024
numbers are also known as squareful, square-full, or 2-full. Paul Erdős and George Szekeres studied such numbers and Solomon W. Golomb named such numbers...
14 KB (1,904 words) - 12:03, 15 October 2024
{\displaystyle 2a-1} . The coloring number of a graph, also known as its Szekeres-Wilf number (Szekeres & Wilf 1968) is always equal to its degeneracy plus 1 (Jensen...
11 KB (1,463 words) - 18:01, 31 December 2023
Made More Relevant to Economics?: An Open Problem, by Eric Maskin The Erdős–Szekeres Problem, by Walter Morris and Valeriu Soltan Novikov’s Conjecture, by...
5 KB (460 words) - 04:03, 13 May 2024
resulting orbit and its analyticity properties are cogently summarized by Szekeres. Several of the solutions are furnished in terms of asymptotic series,...
12 KB (1,188 words) - 18:13, 28 November 2023