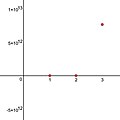
mathematics, tetration (or hyper-4) is an operation based on iterated, or repeated, exponentiation. There is no standard notation for tetration, though Knuth's...

now called hyperoperations. Goodstein also suggested the Greek names tetration, pentation, etc., for the extended operations beyond exponentiation. The...

fifth hyperoperation. Pentation is defined to be repeated tetration, similarly to how tetration is repeated exponentiation, exponentiation is repeated multiplication...

Reuben Goodstein after the Greek prefix of n suffixed with -ation (such as tetration (n = 4), pentation (n = 5), hexation (n = 6), etc.) and can be written...

Wiktionary, the free dictionary. ^^ may refer to: A kaomoji Tetration, the ASCII form of the tetration operator ↑↑ Record separator, control character in the...

in minor details of rounding) and forms an inverse to the operation of tetration. The iterated logarithm is useful in analysis of algorithms and computational...

= 2 × 2 {\displaystyle 2^{2}=2\times 2} . It is also equal to 32 (see tetration). The aliquot sum of 16 is 15, within an aliquot sequence of four composite...

\uparrow 2\uparrow \uparrow (3+2\uparrow \uparrow 8),} which contains three tetrations. In 2019 this was further improved to: N ″ = ( 2 ↑↑ 5138 ) ⋅ ( ( 2 ↑↑...

multiple up arrows, such as ⇈, for iterated, or repeated, exponentiation (tetration). The quantum theory of electron spin uses either upward or downward arrows...

edges to create the Holt graph. 27 is 33, and therefore, it is the second tetration of 3 (23). The 27 club refers to the age when many popular music figures...

by which the magnitude of a googolplex could be represented, such as tetration, hyperoperation, Knuth's up-arrow notation, Steinhaus–Moser notation,...

} This sum is transcendental because it is a Liouville number. Like tetration, there is currently no accepted method of extension of the exponential...

common in computing. The first 21 of them are: Also see Fermat number, Tetration and Hyperoperation § Lower hyperoperations. All of these numbers over...

number, larger than what can be represented even using power towers (tetration). However, it can be represented using layers of Knuth's up-arrow notation...

which makes it a power of two. 256 is 4 raised to the 4th power, so in tetration notation, 256 is 24. 256 is the value of the expression n n {\displaystyle...

the function f ( x ) = x x . {\displaystyle f(x)=x^{x}.} The infinite tetration x x x ⋅ ⋅ ⋅ {\displaystyle x^{x^{x^{\cdot ^{\cdot ^{\cdot }}}}}} or ∞...

exponentiation; this operation is sometimes called hyper-4 or tetration. Iterating tetration leads to another operation, and so on, a concept named hyperoperation...

the set of real or rational numbers, is not commutative or associative. Tetration ( ↑↑ {\displaystyle \uparrow \uparrow } ), as a binary operation on the...

functions, but much more slowly than double exponential functions. However, tetration and the Ackermann function grow faster. See Big O notation for a comparison...

terms, is correct to the first decimal place when n is positive. Also see Tetration: f n(1) = n√2. Using the other fixed point a = f(4) = 4 causes the series...

function is a linear combination of its partial derivatives Euler's infinite tetration theorem – About the limit of iterated exponentiation Euler's rotation...

logit. They are the inverse functions of the double exponential function, tetration, of f(w) = wew, and of the logistic function, respectively. From the perspective...

such a function. This includes addition, multiplication, exponentiation, tetration, etc. The Ackermann function is an example of a function that is not primitive...

H-function Hyper operators Iterated logarithm Pentation Super-logarithms Tetration Lambert W function: Inverse of f(w) = w exp(w). Lamé function Mathieu...

is only around 47 million, the value of S(6) is more than 10⇈15, or in tetration notation, 15 10 {\displaystyle {}^{15}10} , which is equal to 10 ( 10...

{e^{e^{\cdot ^{\cdot ^{e}}}}} _{k\ e'{\text{s}}}=e\uparrow \uparrow k} using tetration or Knuth's up-arrow notation. To see the divergence of the series (4)...

number is too large to reasonably express using exponentiation or even tetration. For more about modern usage for large numbers, see Large numbers. To...

pandigital number 387,420,489 = 196832 = 7293 = 276 = 99 = 318 and in tetration notation 29 387,426,321 = Leyland number using 3 & 18 (318 + 183) 400...

Lambert W function. Some examples of functions that are not elementary: tetration the gamma function non-elementary Liouvillian functions, including the...

hyperoperations, used to build addition, multiplication, exponentiation, tetration, etc. It was studied in 1986 in an investigation involving generalization...