In general relativity, the metric tensor (in this context often abbreviated to simply the metric) is the fundamental object of study. The metric captures...

differential geometry, the Einstein tensor (named after Albert Einstein; also known as the trace-reversed Ricci tensor) is used to express the curvature...

obtained from the Riemann tensor by subtracting a tensor that is a linear expression in the Ricci tensor. In general relativity, the Weyl curvature is the...

tensor fields defined on a Lorentzian manifold representing spacetime. This article is a general description of the mathematics of general relativity...

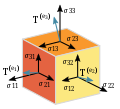
stress-energy tensor The stress–energy tensor, sometimes called the stress–energy–momentum tensor or the energy–momentum tensor, is a tensor physical quantity...

In the mathematical field of differential geometry, a metric tensor (or simply metric) is an additional structure on a manifold M (such as a surface)...

a metric space A metric tensor, in differential geometry, which allows defining lengths of curves, angles, and distances in a manifold Metric tensor (general...

It assigns a tensor to each point of a Riemannian manifold (i.e., it is a tensor field). It is a local invariant of Riemannian metrics that measures...

coordinates Diffusion tensors, the basis of diffusion tensor imaging, represent rates of diffusion in biologic environments In general relativity, four-dimensional...

special relativity and general relativity, a four-tensor is an abbreviation for a tensor in a four-dimensional spacetime. General four-tensors are usually...

quasispherical event horizon. The Kerr metric is an exact solution of the Einstein field equations of general relativity; these equations are highly non-linear...

mathematics, the nonmetricity tensor in differential geometry is the covariant derivative of the metric tensor. It is therefore a tensor field of order three....

stress–energy tensor, the EFE are understood to be equations for the metric tensor gμν, since both the Ricci tensor and scalar curvature depend on the metric in...

In general relativity, a vacuum solution is a Lorentzian manifold whose Einstein tensor vanishes identically. According to the Einstein field equation...

general relativity. Killing tensors satisfy an equation similar to Killing's equation for Killing vectors. Like Killing vectors, every Killing tensor...

relativity is being squashed to zero. The same is true of vector–tensor theories, the deviation of the vector–tensor theories from general relativity...

called a semi-Riemannian manifold, is a differentiable manifold with a metric tensor that is everywhere nondegenerate. This is a generalization of a Riemannian...

1959. Introduction to the mathematics of general relativity Stress–energy tensor Metric tensor (general relativity) Peres, Asher (1959). "Some Gravitational...

{\displaystyle r_{\text{s}}/r} go to zero, the metric becomes the Minkowski metric for special relativity. In practice, the ratio r s / r {\displaystyle...

In Einstein's theory of general relativity, the Schwarzschild metric (also known as the Schwarzschild solution) is an exact solution to the Einstein field...

spacetime. The field tensor was developed by Arnold Sommerfeld after the four-dimensional tensor formulation of special relativity was introduced by Hermann...

formula for the metric tensor g μ ν {\displaystyle g_{\mu \nu }\!} is called the Kerr–Newman metric. It is a generalisation of the Kerr metric for an uncharged...

that the general principle of relativity should also apply to accelerated relative motions, and he used the newly developed tool of tensor calculus to...

In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the canonical pairing of a vector space and its dual. In components...

(electromagnetic tensor, Maxwell tensor, permittivity, magnetic susceptibility, ...), and general relativity (stress–energy tensor, curvature tensor, ...). In...

Like any other Lorentzian spacetime, the Gödel solution represents the metric tensor in terms of a local coordinate chart. It may be easiest to understand...

In mathematics, the signature of a metric tensor g (or equivalently, a real quadratic form thought of as a real symmetric bilinear form on a finite-dimensional...

differential geometry, a tensor density or relative tensor is a generalization of the tensor field concept. A tensor density transforms as a tensor field when passing...

theory of relativity Ricci calculus – Tensor index notation for tensor-based calculations Timeline of gravitational physics and relativity "GW150914:...

manifold) or of the physical space. Tensor fields are used in differential geometry, algebraic geometry, general relativity, in the analysis of stress and...