Karl Theodor Wilhelm Weierstrass (/ˈvaɪərˌstrɑːs, -ˌʃtrɑːs/; German: Weierstraß [ˈvaɪɐʃtʁaːs]; 31 October 1815 – 19 February 1897) was a German mathematician...

In mathematics, the Weierstrass function, named after its discoverer, Karl Weierstrass, is an example of a real-valued function that is continuous everywhere...

specifically in real analysis, the Bolzano–Weierstrass theorem, named after Bernard Bolzano and Karl Weierstrass, is a fundamental result about convergence...

original version of this result was established by Karl Weierstrass in 1885 using the Weierstrass transform. Marshall H. Stone considerably generalized...

linear factors, one for each root. The theorem, which is named for Karl Weierstrass, is closely related to a second result that every sequence tending...

mathematics, the Weierstrass elliptic functions are elliptic functions that take a particularly simple form. They are named for Karl Weierstrass. This class...

the Weierstrass functions are special functions of a complex variable that are auxiliary to the Weierstrass elliptic function. They are named for Karl Weierstrass...

rational number. The theorem is named for Ferdinand von Lindemann and Karl Weierstrass. Lindemann proved in 1882 that eα is transcendental for every non-zero...

{\textstyle t=-\cot(\psi /2).} Weierstrass, Karl (1915) [1875]. "8. Bestimmung des Integrals ...". Mathematische Werke von Karl Weierstrass (in German). Vol. 6....

complex numbers. It is named after the German mathematician Karl Weierstrass (1815–1897). Weierstrass M-test. Suppose that (fn) is a sequence of real- or complex-valued...

after Karl Weierstrass. These include: The Weierstrass approximation theorem, of which one well known generalization is the Stone–Weierstrass theorem...

theorem is used to prove Rolle's theorem. In a formulation due to Karl Weierstrass, this theorem states that a continuous function from a non-empty compact...

Kovalevskaya moved to Berlin, where she began to take private lessons with Karl Weierstrass, since the university would not allow her even to audit classes. He...

named after and first published by Pierre Alphonse Laurent in 1843. Karl Weierstrass had previously described it in a paper written in 1841 but not published...

mathematics, the Weierstrass transform of a function f : R → R {\displaystyle f:\mathbb {R} \to \mathbb {R} } , named after Karl Weierstrass, is a "smoothed"...

German mathematician Karl Weierstrass. Bolzano–Weierstrass theorem Casorati–Weierstrass theorem Weierstrass method Enneper–Weierstrass parameterization...

contemporary philosophy and beyond. Husserl studied mathematics, taught by Karl Weierstrass and Leo Königsberger, and philosophy taught by Franz Brentano and Carl...

the University of Berlin, attending lectures by Leopold Kronecker, Karl Weierstrass and Ernst Kummer. He spent the summer of 1866 at the University of...

the connection between the gamma function and elliptic integrals. Karl Weierstrass further established the role of the gamma function in complex analysis...

received his Ph.D. in mathematics at Berlin, where he studied under Karl Weierstrass. In 1886, he became a professor at the Technische Hochschule Hannover...

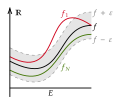
instances of faulty reasoning. The concept, which was first formalized by Karl Weierstrass, is important because several properties of the functions f n {\displaystyle...

century by the seminal work of Bernard Bolzano, Bernhard Riemann, and Karl Weierstrass, and on to the coining of the word fractal in the 20th century with...

physicists Hermann von Helmholtz and Gustav Kirchhoff and mathematician Karl Weierstrass. He wrote that Helmholtz was never quite prepared, spoke slowly, miscalculated...

Kronecker, Kummer and Karl Weierstrass. He received his doctorate (awarded with distinction) in 1870 supervised by Weierstrass. His thesis was on the...

1830s, but the work wasn't published until the 1930s. Like Bolzano, Karl Weierstrass denied continuity of a function at a point c unless it was defined...

at the University of Münster and later wrote his dissertation under Karl Weierstrass and Ernst Kummer at Berlin in 1872. He taught in gymnasia (secondary...

In numerical analysis, the Weierstrass method or Durand–Kerner method, discovered by Karl Weierstrass in 1891 and rediscovered independently by Durand...

and the concept of uniform convergence, and for being the teacher of Karl Weierstrass, who was greatly influenced by Gudermann's course on elliptic functions...

(1894–1967), mathematician Heinrich Martin Weber (1842–1913), mathematician Karl Weierstrass (1815–1897), mathematician Max Zorn (1906–1993), mathematician Heinrich...

German-born tightrope artist Karl Weierstrass (1815–1897), German mathematician Karl Wendlinger (born 1968), Austrian race car driver Karl Williams (born 1971)...