mathematics, the dual quaternions are an 8-dimensional real algebra isomorphic to the tensor product of the quaternions and the dual numbers. Thus, they...
31 KB (4,774 words) - 14:26, 19 November 2024
In mathematics, the quaternion number system extends the complex numbers. Quaternions were first described by the Irish mathematician William Rowan Hamilton...
96 KB (12,689 words) - 12:38, 27 October 2024
subspace. As K-algebras, they generalize the real numbers, complex numbers, quaternions and several other hypercomplex number systems. The theory of Clifford...
64 KB (9,177 words) - 21:08, 17 October 2024
Unit quaternions, known as versors, provide a convenient mathematical notation for representing spatial orientations and rotations of elements in three...
67 KB (11,722 words) - 22:16, 11 November 2024
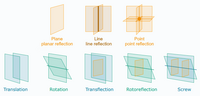
Screw axis (section Dual quaternion)
axis appears in the dual quaternion formulation of a spatial displacement D = ([A], d). The dual quaternion is constructed from the dual vector S = (S, V)...
20 KB (2,103 words) - 09:44, 22 September 2024
of the dual quaternion algebra to 2D geometry. At this present time, the article is focused on a 4-dimensional subalgebra of the dual quaternions which...
10 KB (1,472 words) - 21:12, 30 September 2024
worked with are dual numbers, dual quaternions, and split-biquaternions, all being associative algebras over R. Study's work with dual numbers and line...
16 KB (1,685 words) - 11:26, 18 July 2024
including the axis–angle representation of rotations, the quaternion and dual quaternion representations of rotations and translations, the plücker representation...
34 KB (4,193 words) - 13:28, 11 November 2024
In abstract algebra, the split-quaternions or coquaternions form an algebraic structure introduced by James Cockle in 1849 under the latter name. They...
22 KB (3,199 words) - 01:40, 24 October 2024
Screw theory (redirect from Dual vectors)
dynamics. This is in part because of the relationship between screws and dual quaternions which have been used to interpolate rigid-body motions. Based on screw...
30 KB (4,410 words) - 18:30, 16 November 2024
Biquaternion (redirect from Complex quaternion)
Split-biquaternions when the coefficients are split-complex numbers. Dual quaternions when the coefficients are dual numbers. This article is about the ordinary biquaternions...
23 KB (3,234 words) - 19:23, 21 November 2024
split-biquaternions, while H ⊗ N {\displaystyle H\otimes N} forms dual quaternions. The algebra of dual quaternions is used to express screw displacement, a common mapping...
37 KB (4,225 words) - 22:37, 15 September 2024
Aleksandr Kotelnikov (section Dual quaternions)
dualer Quaternionen auf Kinematik", Annales Academiae Scientiarum Fennicae (1958), 1-13; Gesammelte Werke, volume 2, Applications of dual quaternions...
6 KB (630 words) - 19:16, 6 November 2024
of error is significantly reduced. By formulating the matrices as dual quaternions, it is possible to get a linear equation by which X is solvable in...
8 KB (1,017 words) - 20:12, 25 April 2024
of space science experimental payloads. Hybrid Global Finite-Time Dual-Quaternion Observer and Controller for Velocity-Free Spacecraft Pose Tracking...
10 KB (782 words) - 18:19, 30 April 2024
Alexander McAulay (category Historical treatment of quaternions)
University of Tasmania, Hobart, Tasmania. He was also a proponent of dual quaternions, which he termed "octonions" or "Clifford biquaternions". McAulay was...
6 KB (596 words) - 10:44, 28 April 2024
In mathematics, quaternions are a non-commutative number system that extends the complex numbers. Quaternions and their applications to rotations were...
19 KB (2,230 words) - 16:10, 20 October 2024
{\textbf {q}}^{0}} denote a unit dual quaternion. A homogeneous dual quaternion may be written as a pair of quaternions, Q ^ = Q + ε Q 0 {\displaystyle...
10 KB (1,813 words) - 18:31, 8 August 2023
representing a rotation as numbers in a computer, some people prefer the quaternion representation or the axis+angle representation, because they avoid the...
56 KB (9,991 words) - 17:47, 5 November 2024
TriAx Weight Maps, where many other industry platforms were using Dual Quaternion. This changed in Genesis 3 to allow Daz 3D figures to be more compatible...
8 KB (861 words) - 21:46, 11 August 2024
In algebra, the dual numbers are a hypercomplex number system first introduced in the 19th century. They are expressions of the form a + bε, where a and...
19 KB (2,757 words) - 05:50, 27 October 2024
analysis of movement in machines: Burmester theory Clifford algebra Dual quaternion Euler's rotation theorem Gear ratio Ideal machine Instantaneous center...
8 KB (604 words) - 23:52, 29 July 2024
Biquaternion algebra (category Quaternions)
of quaternion algebras over a field. The biquaternions of William Rowan Hamilton (1844) and the related split-biquaternions and dual quaternions do not...
5 KB (576 words) - 15:47, 21 February 2024
Cross product (section Quaternions)
algebra of quaternions and the non-commutative Hamilton product. In particular, when the Hamilton product of two vectors (that is, pure quaternions with zero...
75 KB (11,474 words) - 18:03, 21 November 2024
of lattices, the dual lattice is a construction analogous to that of a dual vector space. In certain respects, the geometry of the dual lattice of a lattice...
12 KB (1,857 words) - 14:22, 4 October 2024
Rotation matrix (section Quaternion)
unit quaternions. Multiplication of rotation matrices is homomorphic to multiplication of quaternions, and multiplication by a unit quaternion rotates...
99 KB (15,031 words) - 06:23, 11 October 2024
map homomorphism from SU(2) × SU(2) to SO(4) given by quaternion multiplication; see quaternions and spatial rotation. Thus SO(4) is not a simple group...
35 KB (2,368 words) - 05:22, 22 October 2024
September 2010). "Simultaneous robot-world and hand-eye calibration using dual-quaternions and Kronecker product" (PDF). International Journal of the Physical...
40 KB (6,086 words) - 10:33, 12 November 2024
group PSL(2, Z). Ring homographies have been used in quaternion analysis, and with dual quaternions to facilitate screw theory. The conformal group of spacetime...
30 KB (3,641 words) - 01:24, 25 February 2024
described by Thorold Gosset in 1900. The vertices of a dual snub 24-cell are obtained using quaternion simple roots (T') in the generation of the 600 vertices...
6 KB (651 words) - 20:43, 5 January 2024