In vector calculus, a conservative vector field is a vector field that is the gradient of some function. A conservative vector field has the property...
23 KB (3,529 words) - 10:53, 16 March 2025
vector calculus a solenoidal vector field (also known as an incompressible vector field, a divergence-free vector field, or a transverse vector field)...
4 KB (430 words) - 08:36, 28 November 2024
force field F, defined everywhere in space (or within a simply-connected volume of space), is called a conservative force or conservative vector field if...
11 KB (1,646 words) - 12:48, 26 April 2025
In vector calculus and physics, a vector field is an assignment of a vector to each point in a space, most commonly Euclidean space R n {\displaystyle...
28 KB (4,076 words) - 01:44, 23 February 2025
force field is a vector field corresponding with a non-contact force acting on a particle at various positions in space. Specifically, a force field is a...
4 KB (539 words) - 16:03, 20 May 2025
Gradient (redirect from Gradient vector)
In vector calculus, the gradient of a scalar-valued differentiable function f {\displaystyle f} of several variables is the vector field (or vector-valued...
37 KB (5,689 words) - 18:55, 15 July 2025
codomain, Conservative vector field, a vector field that is the gradient of a scalar potential field Hamiltonian vector field, a vector field defined for...
10 KB (2,684 words) - 04:26, 1 June 2025
Vector calculus or vector analysis is a branch of mathematics concerned with the differentiation and integration of vector fields, primarily in three-dimensional...
22 KB (2,135 words) - 04:00, 8 April 2025
In vector calculus, a complex lamellar vector field is a vector field which is orthogonal to a family of surfaces. In the broader context of differential...
9 KB (1,108 words) - 21:08, 13 February 2024
called conservative forces. If the force acting on a body varies over space, then one has a force field; such a field is described by vectors at every...
44 KB (6,112 words) - 01:31, 1 June 2025
Line integral (redirect from Line integral of a vector field)
curve (commonly arc length or, for a vector field, the scalar product of the vector field with a differential vector in the curve). This weighting distinguishes...
21 KB (3,183 words) - 03:16, 18 March 2025
In vector calculus, a Beltrami vector field, named after Eugenio Beltrami, is a vector field in three dimensions that is parallel to its own curl. That...
8 KB (1,062 words) - 01:00, 28 May 2025
Electromagnetic tensor (redirect from Electromagnetic field tensor)
{\displaystyle \phi } is a scalar potential for the irrotational/conservative vector field E → {\displaystyle {\vec {E}}} ) and A → ( x → , t ) {\displaystyle...
18 KB (3,463 words) - 00:28, 25 June 2025
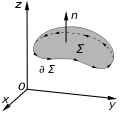
Stokes' theorem (category Vector calculus)
in vector calculus on R 3 {\displaystyle \mathbb {R} ^{3}} . Given a vector field, the theorem relates the integral of the curl of the vector field over...
30 KB (4,860 words) - 18:59, 5 July 2025
if the vector field F is the gradient of some scalar-valued function (i.e., if F is conservative), then F is a path-independent vector field (i.e., the...
20 KB (3,012 words) - 14:30, 10 June 2025
a vector flow can be thought of as a solution to the system of differential equations induced by a vector field. That is, if a (conservative) vector field...
4 KB (593 words) - 09:49, 15 April 2025
any C 1 {\displaystyle C^{1}} vector field that has the path-independence property (so it is a conservative vector field.) must also be irrotational and...
19 KB (2,838 words) - 01:45, 25 February 2025
electric field between atoms is the force responsible for chemical bonding that result in molecules. The electric field is defined as a vector field that...
43 KB (5,520 words) - 08:28, 3 July 2025
justification for belief Conservative force, a physical force whose work is path-independent Conservative vector field, a vector field that is the gradient...
2 KB (280 words) - 23:41, 5 July 2024
exact form. In 3 dimensions, an exact vector field (thought of as a 1-form) is called a conservative vector field, meaning that it is the derivative (gradient)...
15 KB (2,603 words) - 23:11, 2 May 2025
Scalar potential (category Vector calculus)
that V is a scalar potential of the conservative vector field F. Scalar potential is not determined by the vector field alone: indeed, the gradient of a...
15 KB (2,084 words) - 04:51, 6 June 2025
field line is a graphical visual aid for visualizing vector fields. It consists of an imaginary integral curve which is tangent to the field vector at...
15 KB (2,033 words) - 00:48, 10 June 2025
Electric potential (redirect from Vector potential difference)
point can be used. In classical electrostatics, the electrostatic field is a vector quantity expressed as the gradient of the electrostatic potential...
20 KB (2,250 words) - 04:44, 6 June 2025
of which is characterized by an irrotational solenoidal field or a conservative vector field. Control System Analysis: Control Systems - The application...
60 KB (7,200 words) - 19:45, 6 July 2025
conservative vector field this integral evaluates to zero for every closed curve. That means that a line integral between any two points in the field...
9 KB (963 words) - 00:07, 12 May 2025
its reciprocal density (ρ) Particle number (ni) Markov property Conservative vector field Nonholonomic system Equation of state State variable Callen 1985...
9 KB (1,368 words) - 13:50, 26 May 2025
In science, a field is a physical quantity, represented by a scalar, vector, or tensor, that has a value for each point in space and time. An example...
36 KB (4,401 words) - 14:11, 28 June 2025
curl, denoted ∇×, of the vector field vanishes. In the case of the gravitational field g, which can be shown to be conservative, it is equal to the gradient...
9 KB (1,143 words) - 03:16, 22 March 2025
In physics, a gravitational field or gravitational acceleration field is a vector field used to explain the influences that a body extends into the space...
12 KB (1,421 words) - 19:13, 26 April 2025
angle approximation. By the above assumptions the flowfield is Conservative vector field, which means that there exists a perturbation velocity potential...
13 KB (2,043 words) - 18:28, 3 July 2025