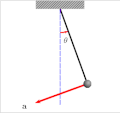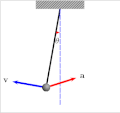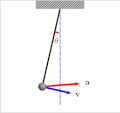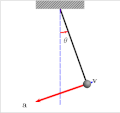
In integral calculus, an elliptic integral is one of a number of related functions defined as the value of certain integrals, which were first studied...

named elliptic functions because they come from elliptic integrals. Those integrals are in turn named elliptic because they first were encountered for the...

 Complete Elliptic integral of  τ 1 X 1 ′ =  Complete Elliptic integral of  1 − τ 1 2 X 2 =  Complete Elliptic integral of  τ 2 X 2 ′ =  Complete Elliptic integral...

In mathematics, the Jacobi elliptic functions are a set of basic elliptic functions. They are found in the description of the motion of a pendulum, as...

to proceed to calculate the elliptic integral. Given Eq. 3 and the Legendre polynomial solution for the elliptic integral: K ( k ) = π 2 ∑ n = 0 ∞ ( (...

using the Arctangent Integral, also called Inverse Tangent Integral. The same procedure also works for the Complete Elliptic Integral of the second kind...

to integrals that generalise the elliptic integrals to all curves over the complex numbers. They include for example the hyperelliptic integrals of type...

/2}{\sqrt {1-x\sin ^{2}\theta }}\ d\theta ,} known as the complete elliptic integral of the second kind, the perimeter can be expressed in terms of that...

which has genus zero: see elliptic integral for the origin of the term. However, there is a natural representation of real elliptic curves with shape invariant...

multivalued function of z {\displaystyle z} . Abelian integrals are natural generalizations of elliptic integrals, which arise when F ( x , w ) = w 2 − P ( x )...

{\displaystyle {\sqrt {1-x^{4}}}} (elliptic integral) 1 ln ⁡ x {\displaystyle {\frac {1}{\ln x}}} (logarithmic integral) e − x 2 {\displaystyle e^{-x^{2}}}...

S2CID 125063457. Prasolov, V.; Solovyev, Y. (1997). Elliptic Functions and Elliptic Integrals. American Mathematical Society. pp. 58–60. ISBN 0-8218-0587-8...

of elliptic integrals are a canonical set of three elliptic integrals to which all others may be reduced. Legendre chose the name elliptic integrals because...

latitude μ, are unrestricted. The above integral is related to a special case of an incomplete elliptic integral of the third kind. In the notation of the...

mathematics, the Carlson symmetric forms of elliptic integrals are a small canonical set of elliptic integrals to which all others may be reduced. They are...

forms: as a relation between complete elliptic integrals, or as a relation between periods and quasiperiods of elliptic functions. The two forms are equivalent...

yksikäsitteisyys (The single-valuedness of the inverse function of the elliptic integral of the first kind). His dissertation was the first and still is the...

using elliptic integrals and jacobi elliptic functions. Smith uses the third fast Jacobi elliptic function estimation algorithm found in the elliptic functions...

quickly, it provides an efficient way to compute elliptic integrals, which are used, for example, in elliptic filter design. The arithmetic–geometric mean...

input x is a non-integer value. Ascending factorial Cahen–Mellin integral Elliptic gamma function Lemniscate constant Pseudogamma function Hadamard's...

circumference of an ellipse can be expressed exactly in terms of the complete elliptic integral of the second kind. More precisely, C e l l i p s e = 4 a ∫ 0 π /...

theta functions and K ( k ) {\displaystyle K(k)} is the complete elliptic integral of the first kind with modulus k {\displaystyle k} shown in the formula...

lemniscate leads to elliptic integrals, as was discovered in the eighteenth century. Around 1800, the elliptic functions inverting those integrals were studied...

Elliptic functions: The inverses of elliptic integrals; used to model double-periodic phenomena. Jacobi's elliptic functions Weierstrass's elliptic functions...

by an incomplete elliptic integral of the second kind, the arc length of a spherical conic is given by an incomplete elliptic integral of the third kind...

one of the Jacobi elliptic functions and K(m) is the complete elliptic integral of the first kind; both are dependent on the elliptic parameter m. The...

with coefficients in finite fields, which amounts to counting integral points on an elliptic curve. An unfinished chapter, consisting of work done during...

the Risch algorithm. other nonelementary integrals, including the Dirichlet integral and elliptic integral. It follows directly from the definition that...

{x^{n+2}+1}}}\,\mathrm {d} x} In the following some Elliptic Integral Singular Values are derived: The elliptic nome function has these important values: q (...

2K(\sin \varphi )}} where K ( k ) {\displaystyle K(k)} is the complete elliptic integral of the first kind K ( k ) = ∫ 0 π / 2 d θ 1 − k 2 sin 2 ⁡ θ . {\displaystyle...