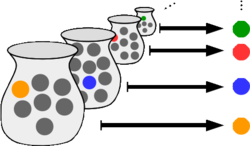
In mathematics, the Hahn decomposition theorem, named after the Austrian mathematician Hans Hahn, states that for any measurable space ( X , Σ ) {\displaystyle...

Lebesgue decomposition theorem provides a way to decompose a measure into two distinct parts based on their relationship with another measure. The theorem states...

Other theorems include: the Hahn decomposition theorem; the Hahn embedding theorem; the Hahn–Kolmogorov theorem; the Hahn–Mazurkiewicz theorem; the Vitali–Hahn–Saks...

In mathematics, Jordan decomposition may refer to Hahn decomposition theorem, and the Jordan decomposition of a measure Jordan normal form of a matrix...

functions Graph decomposition, partition of the edge set of a graph Hahn decomposition theorem of a measure space Jordan decomposition theorem of a signed...

hold, and therefore, μ ( E ) = 0. {\displaystyle \mu (E)=0.} The Hahn decomposition theorem states that for every measurable space ( X , Σ ) {\displaystyle...

the Hahn decomposition theorem is called the Jordan decomposition. The measures μ+, μ− and |μ| are independent of the choice of P and N in the Hahn decomposition...

is the required function. Lebesgue's decomposition theorem shows that the assumptions of the Radon–Nikodym theorem can be found even in a situation which...

analysis) Fubini's theorem (integration) Hahn decomposition theorem (measure theory) Hahn–Kolmogorov theorem (measure theory) Ham sandwich theorem (topology)...

is a theorem in set-theoretic geometry, which states the following: Given a solid ball in three-dimensional space, there exists a decomposition of the...

to this decomposition of a function, one may decompose a signed measure into positive and negative parts — see the Hahn decomposition theorem. Rectifier...

spaces. The spectral theorem also provides a canonical decomposition, called the spectral decomposition, of the underlying vector space on which the operator...

:\Sigma \to [-\infty ,+\infty ]} is a signed measure. Use the Hahn decomposition theorem to write μ = μ + − μ − , {\displaystyle \mu =\mu ^{+}-\mu ^{-}...

the Hopf extension theorem and the Hahn–Kolmogorov extension theorem. Several very similar statements of the theorem can be given. A slightly more involved...

Wold contributed the Cramér–Wold theorem characterizing the normal distribution and developed the Wold decomposition in time series analysis. In microeconomics...

an algebra of sets Hadwiger's theorem – Theorem in integral geometry Hahn decomposition theorem – Measurability theorem Invariant measure – Concept in...

S2CID 119301206. Gowers, Timothy (2010). "Decompositions, approximate structure, transference, and the Hahn–Banach theorem". Bulletin of the London Mathematical...

Partial fraction decomposition over R Vieta's formulas Integer-valued polynomial Algebraic equation Factor theorem Polynomial remainder theorem See also Theory...

more. Hahn series are a further (larger) generalization of Puiseux series, introduced by Hans Hahn in the course of the proof of his embedding theorem in...

as the decomposition of the squared length of a vector into the sum of the squared lengths of several vectors, using the Pythagorean Theorem." The theory...

paradox, the Hahn–Banach theorem, the Banach–Steinhaus theorem, the Banach–Mazur game, the Banach–Alaoglu theorem, and the Banach fixed-point theorem. Stefan...

upper and lower variations to prove the Hahn–Jordan decomposition: according to his version of this theorem, the upper and lower variation are respectively...

This proves the theorem. It follows from the above decomposition that A* is the linear span of states. By the Krein-Milman theorem, the state space of...

to construct the required decomposition of the unit ball in ZF, but also impossible to prove there is no such decomposition. Such statements can be rephrased...

property that is held by all cards). decomposition See tree decomposition, path decomposition, or branch-decomposition. degenerate degeneracy A k-degenerate...

measure μ are finite-valued signed measures. One can apply the Hahn-Jordan decomposition to these measures to split them as μ 1 = μ 1 + − μ 1 − {\displaystyle...

compounds under Otto Hahn and Lise Meitner. He received his Ph.D. at Stuttgart with Prof. Erich Regener for work on the decomposition of ozone. Hellmann's...

primary decomposition, of finitely many primary ideals (which are related to, but not quite the same as, powers of prime ideals). The theorem was first...

algebra problems: QR decomposition (see Householder transformation and Gram–Schmidt decomposition); Singular value decomposition Reduction to Hessenberg...

of the unit ball. With Friedrich Wehrung, Foreman showed that the Hahn–Banach theorem implied the existence of a non-Lebesgue measurable set, even in the...