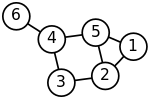
In mathematics, Sperner's lemma is a combinatorial result on colorings of triangulations, analogous to the Brouwer fixed point theorem, which is equivalent...

named after Emanuel Sperner, who published it in 1928. This result is sometimes called Sperner's lemma, but the name "Sperner's lemma" also refers to an...

generalizations, including the Sperner property of a partially ordered set. Sperner's lemma, from 1928, states that every Sperner coloring of a triangulation...

of certain combinatorial structures. For example, in the proofs of Sperner's lemma and the mountain climbing problem the geometric properties of the formula...

local lemma Nakayama's lemma Poincaré's lemma Riesz's lemma Schur's lemma Schwarz's lemma Sperner's lemma Urysohn's lemma Vitali covering lemma Yoneda's...

Knaster, Kuratowski and Mazurkiewicz. The KKM lemma can be proved from Sperner's lemma and can be used to prove the Brouwer fixed-point theorem. Let Δ n −...

{\displaystyle f(P)_{j}\leq P_{j}.} By construction, this is a Sperner coloring. Hence, by Sperner's lemma, there is an n-dimensional simplex whose vertices are...

JSTOR 2320587 Jarvis, Tyler; Tanton, James (2004), "The Hairy Ball Theorem via Sperner's Lemma", American Mathematical Monthly, 111 (7): 599–603, doi:10.1080/00029890...

lemma Littlewood–Offord lemma Pólya–Burnside lemma Sperner's lemma Ky Fan lemma (combinatorial geometry) Berge's lemma Counting lemma Crossing lemma Expander...

Francis Edward (2013), "A Borsuk–Ulam equivalent that directly implies Sperner's lemma", The American Mathematical Monthly, 120 (4): 346–354, doi:10.4169/amer...

constrained. Hall's theorem can be proved (non-constructively) based on Sperner's lemma.: Thm.4.1, 4.2  The theorem has many applications. For example, for...

Lebesgue's number lemma Polytope Simplex Simplicial complex CW complex Manifold Triangulation Barycentric subdivision Sperner's lemma Simplicial approximation...

MR 0183653, S2CID 122892253. Lubell, D. (1966), "A short proof of Sperner's lemma", Journal of Combinatorial Theory, 1 (2): 299, doi:10.1016/S0021-9800(66)80035-2...

protocols are several protocols for envy-free division. They are based on Sperner's lemma. The merits of these protocols is that they put few restrictions on...

the study of fair division problems. Topics in this area include: Sperner's lemma Regular maps A discrete group is a group G equipped with the discrete...

point, but it doesn't describe how to find the fixed point (see also Sperner's lemma). For example, the cosine function is continuous in [−1, 1] and maps...

game with any number of players. Finding a three-colored point in Sperner's Lemma. Finding an envy-free cake-cutting when the utility functions are given...

coloring List coloring List edge-coloring Perfect graph Ramsey's theorem Sperner's lemma Strong coloring Subcoloring Tait's conjecture Total coloring Uniquely...

redirect targets Hairy ball theorem – Theorem in differential topology Sperner's lemma – Theorem on triangulation graph colorings Weisstein, Eric W. "Bolzano's...

Francis Edward (2013), "A Borsuk–Ulam equivalent that directly implies Sperner's lemma", The American Mathematical Monthly, 120 (4): 346–354, doi:10.4169/amer...

upon extending p-adic valuations to the real numbers and extending Sperner's lemma to more general colored graphs. A dissection of a polygon P is a finite...

explained by the degree sum formula. Sperner's lemma is a more advanced application of the same strategy. The lemma states that a certain kind of coloring...

Show that a straight line can contain points of only two colours. Use Sperner's lemma to show that every triangulation of the square into triangles meeting...

Francis Edward (2013), "A Borsuk–Ulam equivalent that directly implies Sperner's lemma", The American Mathematical Monthly, 120 (4): 346–354, doi:10.4169/amer...

 246. ISBN 978-0-521-55846-4. Schaefer, Uwe (3 December 2014). From Sperner's Lemma to Differential Equations in Banach Spaces : An Introduction to Fixed...

1967 and already as an undergraduate published a research paper about Sperner's lemma, which he learned about from Hans Rademacher. He completed his doctorate...

topology now have a combinatorial analog in discrete Morse theory. Sperner's lemma Discrete exterior calculus Topological graph theory Combinatorial topology...

directions, in the case of simplices the combinatorial result known as Sperner's lemma is used to guarantee the existence of an appropriate subsimplex. Once...

and there also exists an EF2 allocation (proof using a variant of Sperner's lemma). For n {\displaystyle n} agents with additive binary valuations (every...

can be reduced to 2D-SPERNER (computing a fully-labeled triangle in a triangulation satisfying the conditions to Sperner's lemma), and therefore it is...