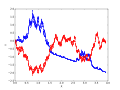
A stochastic differential equation (SDE) is a differential equation in which one or more of the terms is a stochastic process, resulting in a solution...

Stochastic partial differential equations (SPDEs) generalize partial differential equations via random force terms and coefficients, in the same way ordinary...

A backward stochastic differential equation (BSDE) is a stochastic differential equation with a terminal condition in which the solution is required to...

partial differential equations (PDEs) which may be with respect to more than one independent variable, and, less commonly, in contrast with stochastic differential...

In mathematics, a differential equation is an equation that relates one or more unknown functions and their derivatives. In applications, the functions...

dynamical system and differential equation topics, by Wikipedia page. See also list of partial differential equation topics, list of equations. Deterministic...

In physics, a Langevin equation (named after Paul Langevin) is a stochastic differential equation describing how a system evolves when subjected to a combination...

Tsirelson's stochastic differential equation (also Tsirelson's drift or Tsirelson's equation) is a stochastic differential equation which has a weak solution...

In mathematics, stochastic analysis on manifolds or stochastic differential geometry is the study of stochastic analysis over smooth manifolds. It is...

process x t {\displaystyle x_{t}} is defined by the following stochastic differential equation: d x t = − θ x t d t + σ d W t {\displaystyle dx_{t}=-\theta...

convection–diffusion equation is a parabolic partial differential equation that combines the diffusion and convection (advection) equations. It describes physical...

adjoint of the infinitesimal generator of the underlying stochastic process. The Klein–Kramers equation is a special case of that. For a Feller process ( X...

stochastic processes such as Brownian motion (see Wiener process). It has important applications in mathematical finance and stochastic differential equations...

with drift. It is an important example of stochastic processes satisfying a stochastic differential equation (SDE); in particular, it is used in mathematical...

multifractals in turbulence, the stochastic differential equation for growth models for random aggregation (the Kardar–Parisi–Zhang equation) and his groundbreaking...

In mathematics, a partial differential equation (PDE) is an equation which computes a function between various partial derivatives of a multivariable function...

backward stochastic differential equation method is a numerical method that combines deep learning with Backward stochastic differential equation (BSDE)...

stochastic differential equations (QSDE) that are analogous to classical Langevin equations. For the remainder of this article stochastic calculus will...

can be generalized to stochastic systems, in which case the HJB equation is a second-order elliptic partial differential equation. A major drawback, however...

for ordinary differential equations are methods used to find numerical approximations to the solutions of ordinary differential equations (ODEs). Their...

papers developing the field of stochastic calculus, which involves stochastic integrals and stochastic differential equations based on the Wiener or Brownian...

of a stochastic differential equation (SDE). It is an extension of the Euler method for ordinary differential equations to stochastic differential equations...

particular, the theory of stochastic processes. He invented the concept of stochastic integral and stochastic differential equation, and is known as the founder...

of assets—are stochastic. Backward stochastic differential equation Stochastic process Control theory Multiplier uncertainty Stochastic scheduling Definition...

differential equation Cauchy–Euler equation Riccati equation Hill differential equation Gauss–Codazzi equations Chandrasekhar's white dwarf equation Lane-Emden...

random variable Yt : Ω → Rn given by the solution to an Itō stochastic differential equation of the form d Y t = b ( t , Y t ) d t + σ ( t , Y t ) d B t...

backward stochastic differential equation method is a numerical method that combines deep learning with Backward stochastic differential equation (BSDE)...

backward stochastic differential equation method is a numerical method that combines deep learning with Backward stochastic differential equation (BSDE)...

mechanics and information theory, the Fokker–Planck equation is a partial differential equation that describes the time evolution of the probability...

of a stochastic differential equation. It is named after Grigori N. Milstein who first published it in 1974. Consider the autonomous Itō stochastic differential...