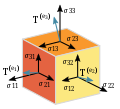
the tensor algebra of a vector space V, denoted T(V) or T•(V), is the algebra of tensors on V (of any rank) with multiplication being the tensor product...

mathematics, the modern component-free approach to the theory of a tensor views a tensor as an abstract object, expressing some definite type of multilinear...

two vectors is sometimes called an elementary tensor or a decomposable tensor. The elementary tensors span V ⊗ W {\displaystyle V\otimes W} in the sense...

mathematics, a tensor is an algebraic object that describes a multilinear relationship between sets of algebraic objects related to a vector space. Tensors may map...

manipulations involving the tensor and wedge symbols. This distinction is developed in greater detail in the article on tensor algebras. Here, there is much...

of scalars. For a commutative ring, the tensor product of modules can be iterated to form the tensor algebra of a module, allowing one to define multiplication...

In multilinear algebra, a tensor contraction is an operation on a tensor that arises from the canonical pairing of a vector space and its dual. In components...

multilinear algebra. Ricci calculus The earliest foundation of tensor theory – tensor index notation. Order of a tensor The components of a tensor with respect...

mathematics, the tensor product of two algebras over a commutative ring R is also an R-algebra. This gives the tensor product of algebras. When the ring...

category of R-algebras. Tensor products The tensor product of two R-algebras is also an R-algebra in a natural way. See tensor product of algebras for more...

algebra concepts find applications in various areas, including: Classical treatment of tensors Dyadic tensor Glossary of tensor theory Metric tensor Bra–ket...

enveloping algebras. The construction proceeds by first building the tensor algebra of the underlying vector space of the Lie algebra. The tensor algebra is simply...

diagonalization. The free algebra generated by V may be written as the tensor algebra ⨁n≥0 V ⊗ ⋯ ⊗ V, that is, the direct sum of the tensor product of n copies...

symmetric algebra. As the symmetric algebra of a vector space is a quotient of the tensor algebra, an element of the symmetric algebra is not a tensor, and...

tensor is antisymmetric with respect to its first three indices. If a tensor changes sign under exchange of each pair of its indices, then the tensor...

In mathematics and physics, a tensor field is a function assigning a tensor to each point of a region of a mathematical space (typically a Euclidean space...

In mathematics, a symmetric tensor is a tensor that is invariant under a permutation of its vector arguments: T ( v 1 , v 2 , … , v r ) = T ( v σ 1 , v...

tensor algebra T ( g ) {\displaystyle T({\mathfrak {g}})} from it. The tensor algebra is a free algebra: it simply contains all possible tensor products...

essentially the composition of functions. In the language of tensor algebra, a particular tensor is associated with a particular shape with many lines projecting...

multilinear algebra, the tensor rank decomposition or rank-R decomposition is the decomposition of a tensor as a sum of R rank-1 tensors, where R is minimal...

relationship between the Ricci tensor and the matter content of the universe. Like the metric tensor, the Ricci tensor assigns to each tangent space of...

notation and manipulation for tensors and tensor fields on a differentiable manifold, with or without a metric tensor or connection. It is also the modern...

In mathematics, the tensor product of representations is a tensor product of vector spaces underlying representations together with the factor-wise group...

"internal" tensor product of a tensor algebra. A tensor algebra comes with a tensor product (the internal one); it can also be equipped with a second tensor product...

category of R-algebras to the category of sets. Free algebras over division rings are free ideal rings. Cofree coalgebra Tensor algebra Free object Noncommutative...

property is used rather than the concrete details. For example, the tensor algebra of a vector space is slightly complicated to construct, but much easier...

Multilinear algebra Tensor Classical treatment of tensors Component-free treatment of tensors Gamas's Theorem Outer product Tensor algebra Exterior algebra Symmetric...

referred to as their tensor product, and can be used to define the tensor algebra. The outer product contrasts with: The dot product (a special case of...

}}i\neq 0\\1,&{\text{if }}i=0\end{cases}}} In linear algebra, it can be thought of as a tensor, and is written δ j i {\displaystyle \delta _{j}^{i}}...

Hopf algebra is particularly nice, since the existence of compatible comultiplication, counit, and antipode allows for the construction of tensor products...