In mathematics, a topological vector space (also called a linear topological space and commonly abbreviated TVS or t.v.s.) is one of the basic structures...

areas of mathematics, a metrizable space is a topological space that is homeomorphic to a metric space. That is, a topological space ( X , τ ) {\displaystyle...

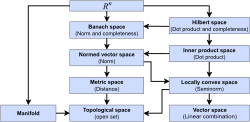
metrizable (resp. pseudometrizable) topological vector space (TVS) is a TVS whose topology is induced by a metric (resp. pseudometric). An LM-space is...

the term "Banach space" and Banach in turn then coined the term "Fréchet space" to mean a complete metrizable topological vector space, without the local...

analysis and related areas of mathematics, a complete topological vector space is a topological vector space (TVS) with the property that whenever points get...

convex topological vector spaces that are completely metrizable (with a choice of complete metric). They are generalizations of Banach spaces, which are...

mathematics, a set in a topological vector space is called bounded or von Neumann bounded, if every neighborhood of the zero vector can be inflated to include...

put it more abstractly every seminormed vector space is a topological vector space and thus carries a topological structure which is induced by the semi-norm...

LF-space – Topological vector space Metrizable topological vector space – A topological vector space whose topology can be defined by a metric Nuclear space –...

if it is complete as a topological vector space. If ( X , τ ) {\displaystyle (X,\tau )} is a metrizable topological vector space (such as any norm induced...

In mathematics, a completely metrizable space (metrically topologically complete space) is a topological space (X, T) for which there exists at least...

mathematics, a barrelled space (also written barreled space) is a topological vector space (TVS) for which every barrelled set in the space is a neighbourhood...

complete metrizable topological vector space X {\displaystyle X} (such as a Fréchet space or an F-space) into a Hausdorff topological vector space Y . {\displaystyle...

on the class of metrizable spaces. Any topological space that is itself finite or countably infinite is separable, for the whole space is a countable dense...

of a paracompact space and a compact space is always paracompact. Every metric space is paracompact. A topological space is metrizable if and only if it...

and related areas of mathematics, a topological property or topological invariant is a property of a topological space that is invariant under homeomorphisms...

a locally convex metrizable topological vector space is a neighborhood of the origin. Closed vector subspaces of bornological space need not be bornological...

metric tensor Metric space – Mathematical space with a notion of distance Metrizable topological vector space – A topological vector space whose topology can...

category theorem is purely topological, it applies to these spaces as well. Completely metrizable spaces are often called topologically complete. However, the...

topology of local convergence in measure is an F-space, i.e. a completely metrizable topological vector space. Moreover, this topology is isometric to global...

In topology and related branches of mathematics, a normal space is a topological space X that satisfies Axiom T4: every two disjoint closed sets of X have...

for a topological space to be a Baire space. (BCT1) Every complete pseudometric space is a Baire space. In particular, every completely metrizable topological...

Conversely, not every topological space can be given a metric. Topological spaces which are compatible with a metric are called metrizable and are particularly...

and topological structures underlie the linear topological space (in other words, topological vector space) structure. A linear topological space is both...

agree in a metric space, but may not be equivalent in other topological spaces. One such generalization is that a topological space is sequentially compact...

type of topological space. For example, every topological manifold is an ANR. Every ANR has the homotopy type of a very simple topological space, a CW complex...

In mathematics, topological groups are the combination of groups and topological spaces, i.e. they are groups and topological spaces at the same time,...

continuous function. Every topological group G {\displaystyle G} (in particular, every topological vector space) becomes a uniform space if we define a subset...

countable dense subset) and if Y {\displaystyle Y} is a metrizable topological vector space then every equicontinuous subset H {\displaystyle H} of L...

mathematics, particularly in functional analysis, a Mackey space is a locally convex topological vector space X such that the topology of X coincides with the Mackey...