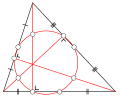
In geometry, a cyclic quadrilateral or inscribed quadrilateral is a quadrilateral (four-sided polygon) whose vertices all lie on a single circle, making...

diametric quadrilateral is a cyclic quadrilateral having one of its sides as a diameter of the circumcircle. A Hjelmslev quadrilateral is a quadrilateral with...

century Indian mathematician, is used to find the area of any convex cyclic quadrilateral (one that can be inscribed in a circle) given the lengths of the...

of quadrilaterals are inscriptable quadrilateral, inscriptible quadrilateral, inscribable quadrilateral, circumcyclic quadrilateral, and co-cyclic quadrilateral...

relation between the four sides and two diagonals of a cyclic quadrilateral (a quadrilateral whose vertices lie on a common circle). The theorem is named...

that the centers of the incircles of certain triangles inside a cyclic quadrilateral are vertices of a rectangle. It was originally stated on a sangaku...

not necessarily concyclic. After triangles, the special case of cyclic quadrilaterals has been most extensively studied. In general the centre O of a...

complete quadrilaterals that are associated with cyclic quadrilaterals, based on the work of Barbu and Patrascu. Given any cyclic quadrilateral ABCD, let...

theorem for cyclic quadrilaterals states that the incentres of the four triangles determined by the vertices of a cyclic quadrilateral taken three at a...

of the diagonal triangle of a cyclic quadrilateral. The point of intersection of the bimedians of the cyclic quadrilateral belongs to the nine-point circle...

result in geometry is his formula for cyclic quadrilaterals. Given the lengths of the sides of any cyclic quadrilateral, Brahmagupta gave an approximate and...

called the circumscribed circle, is called a cyclic polygon, or in the special case n = 4, a cyclic quadrilateral. All rectangles, isosceles trapezoids, right...

special case of Brahmagupta's formula (for the case of a degenerate cyclic quadrilateral). A modern proof, which uses algebra and is quite different from...

these quadrilaterals. A harmonic quadrilateral is a quadrilateral that can be inscribed in a circle (a cyclic quadrilateral) and in which the products of...

bisector. The internal angle bisectors of a convex quadrilateral either form a cyclic quadrilateral (that is, the four intersection points of adjacent...

bicentric quadrilaterals have all the properties of both tangential quadrilaterals and cyclic quadrilaterals. Other names for these quadrilaterals are chord-tangent...

concyclic points. All triangles are cyclic polygons. Cyclic quadrilateral, a special case of a cyclic polygon. Smallest-circle problem, the related problem...

five-volume work Treatise on the Quadrilateral. A generalization of the law of tangents holds for a cyclic quadrilateral ◻ A B C D . {\displaystyle \square...

triangulates a cyclic quadrilateral, the sum of inradii of triangles is constant. After proving the quadrilateral case, the general case of the cyclic polygon...

tangential quadrilateral are given in Tangential quadrilateral#Collinear points. In a cyclic quadrilateral, the circumcenter, the vertex centroid (the intersection...

the sides of the quadrilateral are the vertices of a cyclic quadrilateral. A convex quadrilateral is orthodiagonal if and only if its Varignon parallelogram...

example is the cyclic quadrilateral. Every regular polygon and every triangle is a cyclic polygon. A polygon that is both cyclic and tangential is called...

regarded as a quadrilateral with one side of length zero. From this perspective, as d {\displaystyle d} approaches zero, a cyclic quadrilateral converges...

general quadrilateral. It works on both convex and concave quadrilaterals, whether it is cyclic or not. The formula also works on crossed quadrilaterals provided...

area can be computed using Brahmagupta's formula for the area of a cyclic quadrilateral, which with two sides equal simplifies to K = ( s − c ) ( s − a )...

consequence of the theorem, opposite angles of cyclic quadrilaterals sum to 180°; conversely, any quadrilateral for which this is true can be inscribed in...

his famous theorem on the diagonals of a cyclic quadrilateral: Brahmagupta's theorem: If a cyclic quadrilateral has diagonals that are perpendicular to...

constructing a cyclic quadrilateral inside the Lexell circle, using the property that pairs of opposite angles in a spherical cyclic quadrilateral have the...

theorem on the diagonals of a cyclic quadrilateral. Chapter 12 also included a formula for the area of a cyclic quadrilateral (a generalization of Heron's...

his famous theorem on the diagonals of a cyclic quadrilateral: Brahmagupta's theorem: If a cyclic quadrilateral has diagonals that are perpendicular to...