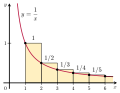
field of real analysis, the monotone convergence theorem is any of a number of related theorems proving the good convergence behaviour of monotonic sequences...

martingale convergence theorem is a random variable analogue of the monotone convergence theorem, which states that any bounded monotone sequence converges. There...

rules and bargaining systems. Monotone class theorem, in measure theory Monotone convergence theorem, in mathematics Monotone polygon, a property of a geometric...

sufficient condition for the convergence of expected values of random variables. Lebesgue's dominated convergence theorem. Let ( f n ) {\displaystyle (f_{n})}...

there exists a monotone subsequence, likewise also bounded. It follows from the monotone convergence theorem that this subsequence converges. The general...

f dμ ≤ dν. The supremum of all such functions, along with the monotone convergence theorem, then furnishes the Radon–Nikodym derivative. The fact that the...

(following from Brouwer's bar theorem) and is strong enough to give short proofs of key theorems. The monotone convergence theorem (described as the fundamental...

take limits under the integral sign (via the monotone convergence theorem and dominated convergence theorem). While the Riemann integral considers the area...

on N {\displaystyle N} . Fatou's lemma does not require the monotone convergence theorem, but the latter can be used to provide a quick and natural proof...

mathematics, the integral test for convergence is a method used to test infinite series of monotonic terms for convergence. It was developed by Colin Maclaurin...

convergence results specify exact conditions which allow one to interchange limits and expectations, as specified below. Monotone convergence theorem:...

it follows from the monotone convergence theorem for series that the sum of this infinite series is equal to e. The binomial theorem is closely related...

monotonically decreasing sequence S2m+1, the monotone convergence theorem then implies that this sequence converges as m approaches infinity. Similarly, the...

mathematical field of analysis, Dini's theorem says that if a monotone sequence of continuous functions converges pointwise on a compact space and if the...

n → ∞ c n = L {\displaystyle \lim _{n\to \infty }c_{n}=L} . (Monotone convergence theorem) If a n {\displaystyle a_{n}} is bounded and monotonic for all...

In mathematics, a monotonic function (or monotone function) is a function between ordered sets that preserves or reverses the given order. This concept...

prove the central limit theorem, but also to provide bounds on the rates of convergence for selected metrics. The convergence to the normal distribution...

theorem, the Stone-Weierstrass theorem, Fatou's lemma, and the monotone convergence and dominated convergence theorems. Various ideas from real analysis...

_{s=0}^{\infty }|X_{s+1}-X_{s}|\cdot \mathbf {1} _{\{\tau >s\}}} . By the monotone convergence theorem E [ M ] = E [ | X 0 | ] + ∑ s = 0 ∞ E [ | X s + 1 − X s | ⋅ 1...

follows using the monotone convergence theorem. By assumption (3), the infinite sequence on the right-hand side of (15) converges, hence SN is integrable...

Mohr–Mascheroni theorem (geometry) Monge's theorem (geometry) Monodromy theorem (complex analysis) Monotone class theorem (measure theory) Monotone convergence theorem...

functions on ( X , Σ ) {\displaystyle (X,\Sigma )} , by Lebesgue's monotone convergence theorem ν {\displaystyle \nu } can be shown to correspond to an L 1 (...

Vitali convergence theorem Fichera convergence theorem Cafiero convergence theorem Fatou's lemma Monotone convergence theorem for integrals (Beppo Levi's lemma)...

contained in the bounded set C 0 {\displaystyle C_{0}} . The monotone convergence theorem for bounded sequences of real numbers now guarantees the existence...

{\displaystyle p<\infty ,} the Minkowski inequality and the monotone convergence theorem imply that ∫ ( ∑ n = 0 ∞ | u n | ) p d μ ≤ ( ∑ n = 0 ∞ ‖ u n...

In mathematics, the Stolz–Cesàro theorem is a criterion for proving the convergence of a sequence. It is named after mathematicians Otto Stolz and Ernesto...

integral Lebesgue integration Monotone convergence theorem – relates monotonicity with convergence Intermediate value theorem – states that for each value...

Fatou's lemma and the monotone convergence theorem hold if almost everywhere convergence is replaced by (local or global) convergence in measure. If μ is...

monotone convergence theorem Fatou's lemma Absolutely continuous Uniform absolute continuity Total variation Radon–Nikodym theorem Fubini's theorem Double...

sequences and modes of convergence, and different proof techniques may be more appropriate than others for proving each type of convergence of each type of sequence...