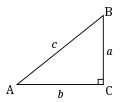
In Euclidean geometry, the Erdős–Mordell inequality states that for any triangle ABC and point P inside ABC, the sum of the distances from P to the sides...

Louis Joel Mordell (28 January 1888 – 12 March 1972) was an American-born British mathematician, known for pioneering research in number theory. He was...

Castelnuovo–Severi inequality Cheng's eigenvalue comparison theorem Clifford's theorem on special divisors Cohn-Vossen's inequality Erdős–Mordell inequality Euler's...

Copeland–Erdős constant Erdős–Tenenbaum–Ford constant Erdős–Bacon number Erdős–Borwein constant Erdős–Diophantine graph Erdős–Mordell inequality Chung–Erdős inequality...

this ratio is as small as 2. This is the Erdős–Mordell inequality; a stronger variant of it is Barrow's inequality, which replaces the perpendicular distances...

{\displaystyle PA\cdot PB\cdot PC\geq (PD+PE)(PE+PF)(PF+PD).} Further, the Erdős–Mordell inequality states that P A + P B + P C P D + P E + P F ≥ 2 {\displaystyle...

this inequality was published in 1937, as his solution to a problem posed in the American Mathematical Monthly of proving the Erdős–Mordell inequality. This...

Equilateral triangle Euler's line Euler's theorem in geometry Erdős–Mordell inequality Exeter point Exterior angle theorem Fagnano's problem Fermat point...

chapter on inequalities includes the Erdős–Mordell inequality on sums of distances from the sides of a triangle and Weitzenböck's inequality relating the...

the Erdős–Ko–Rado theorem limits the number of sets in a family of sets for which every two sets have at least one element in common. Paul Erdős, Chao...

before his death, D. K. Kazarinoff provided a simple proof of the Erdős-Mordell inequality for triangles and gave a generalization to three dimensions. Nicholas...

(combinatorics) Erdős–Ginzburg–Ziv theorem Polynomial method Van der Waerden's theorem Szemerédi's theorem Collatz conjecture Gilbreath's conjecture Erdős–Graham...

(ergodic theory) Erdős–Anning theorem (discrete geometry) Erdős–Dushnik–Miller theorem (set theory) Erdős–Gallai theorem (graph theory) Erdős–Ginzburg–Ziv...

professor in 1923, and chaired the mathematics department in 1944–1945. Erdős, Paul; Mordell, L. J.; Barrow, David F. (1937), "Solution to problem 3740", American...

congruent numbers. Erdős–Moser problem: is 1 1 + 2 1 = 3 1 {\displaystyle 1^{1}+2^{1}=3^{1}} the only solution to the Erdős–Moser equation? Erdős–Straus conjecture:...

numbers. The Mordell conjecture (already proven in general by Gerd Faltings). As equivalent, Vojta's conjecture in dimension 1. The Erdős–Woods conjecture...

additive basis of degree 4. (About an open problem for additive bases, see Erdős–Turán conjecture on additive bases.) Historically the theorems above were...

negation, and are thus theorems. In their discussion of the Hecke, Deuring, Mordell, Heilbronn theorem, Ireland & Rosen (1990, p. 359) say The method of proof...

appointment at the University of Manchester in 1937, just at the time when Louis Mordell had recruited émigrés from continental Europe to build an outstanding department...

conjecture and Beal's conjecture, am + bn = ck with inequality restrictions on the exponents the Erdős–Moser equation, 1k + 2k + ⋯ + (m – 1)k = mk A general...

(2): 239–254. doi:10.1307/mmj/1339011525. ISSN 0026-2285. Mahler, Kurt; Mordell, Louis Joel (4 June 1968). "Applications of a theorem by A. B. Shidlovski"...

NT]. Booker, Andrew R.; Sutherland, Andrew V. (2021). "On a question of Mordell". Proceedings of the National Academy of Sciences. 118 (11). arXiv:2007...

results in this paper, the most famous of which is the first proof of the Mordell conjecture (a conjecture dating back to 1922). Other theorems proved in...

arithmetic algebraic geometry, he received medal primarily for his proof of the Mordell Conjecture." Michael Freedman University of California, San Diego, US Microsoft...

atmospheres, theory of relativity and the interior structure of stars. Louis Mordell, contributions in number theory. Bernhard Neumann, contributions to group...