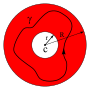
mathematics, the Laurent series of a complex function f ( z ) {\displaystyle f(z)} is a representation of that function as a power series which includes...
16 KB (2,675 words) - 20:24, 29 December 2024
power series Puiseux series are an extension of formal Laurent series, allowing fractional exponents Rational series Ring of restricted power series The...
53 KB (10,048 words) - 13:06, 29 January 2025
Principal part (section Laurent series definition)
independent meanings but usually refers to the negative-power portion of the Laurent series of a function. The principal part at z = a {\displaystyle z=a} of a...
2 KB (283 words) - 08:17, 21 January 2025
be calculated by finding Laurent series expansions, and one can define the residue as the coefficient a−1 of a Laurent series. The concept can be used...
15 KB (3,101 words) - 12:03, 13 December 2024
formal power series is also a differential algebra, with differentiation performed term-by-term. Laurent series generalize power series by admitting terms...
78 KB (12,802 words) - 13:15, 22 January 2025
Look up Laurent or laurent in Wiktionary, the free dictionary. Laurent may refer to: Laurent (name), a French masculine given name and a surname Saint...
742 bytes (131 words) - 10:26, 5 March 2024
Maclaurin series of f is its Taylor series about x 0 = 0 {\displaystyle x_{0}=0} . A Laurent series is a generalization of the Taylor series, allowing...
9 KB (968 words) - 14:45, 29 December 2024
Infinitesimal (section Laurent series)
of Dales and Woodin. Since a Taylor series evaluated with a Laurent series as its argument is still a Laurent series, the system can be used to do calculus...
37 KB (5,092 words) - 16:24, 9 February 2025
variable x; see Laurent series. For example, f (x) = e−1/x2 can be written as a Laurent series. The generalization of the Taylor series does converge to...
48 KB (8,229 words) - 01:10, 29 January 2025
Riemann zeta function (redirect from Series of reciprocal powers)
of order one at s = 1. It can therefore be expanded as a Laurent series about s = 1; the series development is then ζ ( s ) = 1 s − 1 + ∑ n = 0 ∞ γ n n...
74 KB (11,140 words) - 04:53, 19 February 2025
Alphonse Laurent (18 July 1813 – 2 September 1854) was a French mathematician, engineer, and Military Officer best known for discovering the Laurent series, an...
4 KB (345 words) - 12:36, 29 July 2024
Laurent series Auguste Laurent (1807–1853), French chemist Christian Laurent, French engineer Joseph Jean Pierre Laurent, a French astronomer Laurent...
5 KB (580 words) - 13:39, 9 October 2024
x^{-1}+1+x^{1}+x^{2}+\cdots } is not considered a power series (although it is a Laurent series). Similarly, fractional powers such as x 1 2 {\textstyle...
19 KB (3,329 words) - 13:46, 23 January 2025
Puiseux series becomes a Laurent series in an nth root of the indeterminate. For example, the example above is a Laurent series in x 1 / 6 . {\displaystyle...
32 KB (5,535 words) - 22:01, 11 July 2024
Hurwitz zeta function (section Laurent series)
powers of integers. The Laurent series expansion can be used to define generalized Stieltjes constants that occur in the series ζ ( s , a ) = 1 s − 1 +...
22 KB (4,220 words) - 10:29, 14 August 2024
Qa,t(z) is solved from the product of P by the principal part of the Laurent series of f at a: It is proportional to the relevant Frobenius covariant. Then...
55 KB (10,417 words) - 10:59, 6 February 2025
Residue theorem (section Series methods)
residue Res(f, c) of f at c is the coefficient a−1 of (z − c)−1 in the Laurent series expansion of f around c. Various methods exist for calculating this...
13 KB (3,284 words) - 09:31, 29 January 2025
power series (in which k ≥ 0). Since any Laurent series is a fraction of a power series divided by a power of x (as opposed to an arbitrary power series),...
87 KB (10,305 words) - 04:18, 15 February 2025
(ln) from BetterExplained "Taylor series of 1 / (1 - r/200)". WolframAlpha. Retrieved January 3, 2025. "Laurent series of ln(2) / ln(1 + r/100)". WolframAlpha...
12 KB (1,806 words) - 06:19, 9 January 2025
Bernoulli number (section Laurent series)
&0<&|x|<\pi .\end{aligned}}} The Bernoulli numbers appear in the following Laurent series: Digamma function: ψ ( z ) = ln z − ∑ k = 1 ∞ B k + k z k {\displaystyle...
93 KB (13,028 words) - 19:46, 10 February 2025
his performance in Red Pitch. In June 2023, Lovehall plays Laurent in musical television series Champion by Candice Carty-Williams, for BBC One. In March...
7 KB (408 words) - 09:44, 22 February 2025
In mathematics, a Laurent polynomial (named after Pierre Alphonse Laurent) in one variable over a field F {\displaystyle \mathbb {F} } is a linear combination...
4 KB (672 words) - 04:09, 10 December 2024
function is meromorphic. Many important tools of complex analysis such as Laurent series and the residue theorem require that all relevant singularities of the...
4 KB (567 words) - 14:43, 22 January 2024
cosine series Fourier transform Gibbs phenomenon Half range Fourier series Laurent series – the substitution q = eix transforms a Fourier series into a...
74 KB (11,694 words) - 17:59, 24 February 2025
conjugation), then the resulting ring of Laurent series is a noncommutative division ring known as a skew Laurent series ring; if σ = id then it features the...
12 KB (1,482 words) - 06:05, 20 February 2025
{\displaystyle z_{0},} a nonzero meromorphic function f is the sum of a Laurent series with at most finite principal part (the terms with negative index values):...
9 KB (1,479 words) - 15:16, 16 June 2024
normalized valuation. The formal Laurent series over a finite field: the ring of integers of Fq((T)) is the ring of formal power series Fq[[T]]. Its maximal ideal...
11 KB (1,661 words) - 17:28, 15 January 2025
Euler's constant (section Series expansions)
x-\gamma } . Evaluations of the digamma function at rational values. The Laurent series expansion for the Riemann zeta function*, where it is the first of the...
71 KB (9,550 words) - 01:29, 13 January 2025
the context of Laurent polynomials and Laurent series, the exponents of a monomial may be negative, and in the context of Puiseux series, the exponents...
10 KB (1,596 words) - 09:47, 12 November 2024
the solution of known regions in Laurent series (about r = ∞ {\displaystyle r=\infty } ), instead of Taylor series (about r = 0 {\displaystyle r=0} )...
33 KB (5,069 words) - 00:29, 16 December 2024