In mathematics, the simplex category (or simplicial category or nonempty finite ordinal category) is the category of non-empty finite ordinals and order-preserving...
4 KB (515 words) - 14:51, 15 January 2023
Simplicial set (redirect from Category of simplicial sets)
homotopy category of topological spaces. Formally, a simplicial set may be defined as a contravariant functor from the simplex category to the category of sets...
23 KB (3,384 words) - 09:16, 24 April 2025
In mathematics, a category (sometimes called an abstract category to distinguish it from a concrete category) is a collection of "objects" that are linked...
21 KB (2,525 words) - 18:54, 19 March 2025
Category theory is a general theory of mathematical structures and their relations. It was introduced by Samuel Eilenberg and Saunders Mac Lane in the...
34 KB (3,910 words) - 19:56, 5 July 2025
closed. The simplex category can be used to construct an example of non-symmetric compact closed category. The simplex category is the category of non-zero...
9 KB (1,678 words) - 08:55, 26 October 2024
In mathematics, higher category theory is the part of category theory at a higher order, which means that some equalities are replaced by explicit arrows...
9 KB (1,016 words) - 14:35, 30 April 2025
In category theory, a branch of mathematics, a pushout (also called a fibered coproduct or fibered sum or cocartesian square or amalgamated sum) is the...
13 KB (1,984 words) - 23:29, 23 June 2025
Functor (redirect from Functor (category theory))
In mathematics, specifically category theory, a functor is a mapping between categories. Functors were first considered in algebraic topology, where algebraic...
24 KB (3,550 words) - 22:28, 25 April 2025
In category theory, a branch of mathematics, the opposite category or dual category C op {\displaystyle C^{\text{op}}} of a given category C {\displaystyle...
5 KB (619 words) - 08:01, 2 May 2025
0-dimensional simplex is a point, a 1-dimensional simplex is a line segment, a 2-dimensional simplex is a triangle, a 3-dimensional simplex is a tetrahedron...
51 KB (7,872 words) - 18:17, 21 June 2025
In mathematics, a monoidal category (or tensor category) is a category C {\displaystyle \mathbf {C} } equipped with a bifunctor ⊗ : C × C → C {\displaystyle...
18 KB (2,436 words) - 07:41, 19 June 2025
In category theory, a branch of mathematics, a pullback (also called a fiber product, fibre product, fibered product or Cartesian square) is the limit...
16 KB (2,061 words) - 08:26, 24 June 2025
In category theory, a branch of mathematics, the abstract notion of a limit captures the essential properties of universal constructions such as products...
27 KB (4,333 words) - 16:33, 22 June 2025
Morphism (redirect from Morphism (category theory))
In mathematics, a morphism is a concept of category theory that generalizes structure-preserving maps such as homomorphism between algebraic structures...
13 KB (1,664 words) - 22:58, 3 July 2025
In category theory, a branch of mathematics, duality is a correspondence between the properties of a category C and the dual properties of the opposite...
5 KB (753 words) - 01:33, 3 June 2025
specifically category theory, a quasi-category (also called quasicategory, weak Kan complex, inner Kan complex, infinity category, ∞-category, Boardman complex...
22 KB (3,351 words) - 12:35, 11 June 2025
specifically in category theory, a preadditive category is another name for an Ab-category, i.e., a category that is enriched over the category of abelian...
12 KB (1,652 words) - 15:51, 6 May 2025
precisely a simple object in the category of (say left) modules. simplex category The simplex category Δ is the category where an object is a set [n] =...
78 KB (11,821 words) - 20:01, 5 July 2025
Applied category theory Category of sets Concrete category Category of vector spaces Category of graded vector spaces Category of chain complexes Category of...
5 KB (402 words) - 15:20, 29 March 2024
} f)(x) ≃ F(g)(F(f)(x)), where x ≃ y means x ≤ y and y ≤ x. FinOrd Simplex category Eklund et al. 2018, Section 1.3. "PreOrd in nLab". ncatlab.org. Clementino...
4 KB (397 words) - 19:22, 28 May 2025
For example, by definition, a simplicial set is a presheaf on the simplex category Δ and a representable simplicial set is exactly of the form Δ n = Hom...
4 KB (798 words) - 09:09, 23 April 2025
nerve N h c ( C ) {\displaystyle N^{hc}(C)} of a 2-category C is a simplicial set where each n-simplex is determined by the following data: n objects x...
19 KB (2,524 words) - 10:33, 29 April 2025
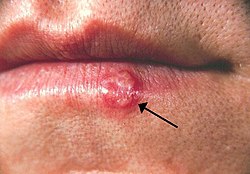
Herpes (redirect from Herpes Simplex)
Herpes simplex, often known simply as herpes, is a viral infection caused by the herpes simplex virus. Herpes infections are categorized by the area of...
82 KB (8,901 words) - 03:40, 12 July 2025
Applied category theory is an academic discipline in which methods from category theory are used to study other fields including but not limited to computer...
8 KB (683 words) - 18:20, 25 June 2025
In mathematics, specifically in category theory, a pre-abelian category is an additive category that has all kernels and cokernels. Spelled out in more...
10 KB (1,382 words) - 03:45, 26 March 2024
In category theory, a category is Cartesian closed if, roughly speaking, any morphism defined on a product of two objects can be naturally identified...
18 KB (2,611 words) - 01:50, 26 March 2025
the mathematical field of category theory, the product of two categories C and D, denoted C × D and called a product category, is an extension of the concept...
3 KB (323 words) - 11:46, 11 May 2023
Yoneda lemma (category Lemmas in category theory)
In mathematics, the Yoneda lemma is a fundamental result in category theory. It is an abstract result on functors of the type morphisms into a fixed object...
20 KB (3,448 words) - 09:53, 27 May 2025
In category theory, a branch of mathematics, a functor category D C {\displaystyle D^{C}} is a category where the objects are the functors F : C → D {\displaystyle...
11 KB (1,776 words) - 18:31, 16 May 2025
simplicial sets, which are presheaves on the simplex category). If C is a model category, then so is the category Pro(C) of pro-objects in C. However, a model...
18 KB (2,402 words) - 23:20, 25 April 2025