the Szilassi polyhedron is a nonconvex polyhedron, topologically a torus, with seven hexagonal faces. The 14 vertices and 21 edges of the Szilassi polyhedron...

is seven. The Császár polyhedron has the fewest possible vertices of any embedded toroidal polyhedron, and the Szilassi polyhedron has the fewest possible...

was soon dubbed the Szilassi polyhedron. This is mathematically significant because the tetrahedron and the Szilassi polyhedron are the only two known...

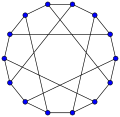
realizations. An example is the Szilassi polyhedron, a toroidal polyhedron that realizes the Heawood map. In this case, the polyhedron is much less symmetric than...

polyhedron is named after Hungarian topologist Ákos Császár, who discovered it in 1949. The dual to the Császár polyhedron, the Szilassi polyhedron,...

alongside other uniform prisms, has 14 faces. The Szilassi polyhedron and its dual, the Császár polyhedron, are the simplest toroidal polyhedra; they have...

and so on. One particularly interesting example is the Szilassi polyhedron, a Toroidal polyhedron with 7 non-convex six sided faces. Frank Chester. "The...

Rhombohedron Scalenohedron Schönhardt polyhedron Square bifrustum Square truncated trapezohedron Szilassi polyhedron Tetradecahedron Tetradyakis hexahedron...

square hosohedron is another polyhedron with four faces, but it does not have triangular faces. The Szilassi polyhedron and the tetrahedron are the only...

Rhombohedron Scalenohedron Schönhardt polyhedron Square bifrustum Square truncated trapezohedron Szilassi polyhedron Tetradecahedron Tetradyakis hexahedron...

upper bound of 7 is sharp: certain toroidal polyhedra such as the Szilassi polyhedron require seven colors. A Möbius strip requires six colors (Tietze...

maximal. The map can be faithfully realized as the Szilassi polyhedron, the only known polyhedron apart from the tetrahedron such that every pair of faces...

Rhombohedron Scalenohedron Schönhardt polyhedron Square bifrustum Square truncated trapezohedron Szilassi polyhedron Tetradecahedron Tetradyakis hexahedron...

forms an embedding of the Heawood graph onto the torus. Grünbaum, Branko; Szilassi, Lajos (2009), "Geometric Realizations of Special Toroidal Complexes",...

Szemerédi's theorem according to ergodic theory. Lajos Szilassi discovers the Szilassi polyhedron. Joel L. Weiner describes a version of the tennis ball...

Rhombohedron Scalenohedron Schönhardt polyhedron Square bifrustum Square truncated trapezohedron Szilassi polyhedron Tetradecahedron Tetradyakis hexahedron...

triacontahedron. The second edition also includes the Császár polyhedron and Szilassi polyhedron, toroidal polyhedra with non-regular faces but with pairwise...

Differential Geometry. 6 (3): 271–283. doi:10.4310/jdg/1214430493. MR 0314057. Szilassi, Lajos (2008). "A polyhedral model in Euclidean 3-space of the six-pentagon...