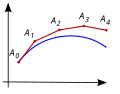
The Petrov–Galerkin method is a mathematical method used to approximate solutions of partial differential equations which contain terms with odd order...

Bubnov–Galerkin method can be viewed as applying an orthogonal projection to the operator. Petrov–Galerkin method (after Georgii I. Petrov) allows using...

The streamline upwind Petrov–Galerkin pressure-stabilizing Petrov–Galerkin formulation for incompressible Navier–Stokes equations can be used for finite...

finite element method The Petrov–Galerkin method The streamline upwind Petrov–Galerkin method (SUPG) The Discontinuous Galerkin method Jewish Encyclopedia...

In mathematics and computational science, the Euler method (also called the forward Euler method) is a first-order numerical procedure for solving ordinary...

The milestone of stabilized methods for this class of problems can be considered the Streamline Upwind Petrov-Galerkin method (SUPG), designed during 80s...

as: Petrov-Galerkin Method: This method is similar to the Bubnov-Galerkin approach but differs in the choice of test functions. In the Petrov-Galerkin method...

In numerical analysis, finite-difference methods (FDM) are a class of numerical techniques for solving differential equations by approximating derivatives...

pass arbitrary-order patch tests, based on a Petrov–Galerkin method. One recent advance in meshfree methods aims at the development of computational tools...

recent schemes, the Discontinuous Galerkin method, Hybrid Mixed Mimetic method, the Nodal Mimetic Finite Difference method, some Discrete Duality Finite Volume...

approximation in this process, the finite element method is commonly introduced as a special case of Galerkin method. The process, in mathematical language, is...

Runge–Kutta methods (English: /ˈrʊŋəˈkʊtɑː/ RUUNG-ə-KUUT-tah) are a family of implicit and explicit iterative methods, which include the Euler method, used...

"Large deformation analysis of elastic bodies by nonlinear Petrov–Galerkin natural element method". Advances in Mechanical Engineering. 11 (4): 168781401984629...

In numerical analysis, the Crank–Nicolson method is a finite difference method used for numerically solving the heat equation and similar partial differential...

element method, discontinuous Galerkin finite element method (DGFEM), element-free Galerkin method (EFGM), interpolating element-free Galerkin method (IEFGM)...

In mathematics, the power series method is used to seek a power series solution to certain differential equations. In general, such a solution assumes...

variation of parameters, also known as variation of constants, is a general method to solve inhomogeneous linear ordinary differential equations. For first-order...

In mathematics, the method of undetermined coefficients is an approach to finding a particular solution to certain nonhomogeneous ordinary differential...

In mathematics, the method of characteristics is a technique for solving partial differential equations. Typically, it applies to first-order equations...

mathematics, separation of variables (also known as the Fourier method) is any of several methods for solving ordinary and partial differential equations, in...

an implicit solution which involves a nonelementary integral. This same method is used to solve the period of a simple pendulum. Integrating factors are...

In mathematics, the Schwarz alternating method or alternating process is an iterative method introduced in 1869–1870 by Hermann Schwarz in the theory of...

sequences: the first for sequences of iterations of an iterative numerical method and the second for sequences of successively more accurate numerical discretizations...

The finite volume method (FVM) is a method for representing and evaluating partial differential equations in the form of algebraic equations. In the finite...

used the Method of exhaustion of Eudoxus. In medieval Europe the quadrature meant calculation of area by any method. More often the Method of indivisibles...

differential equation method is a numerical method that combines deep learning with Backward stochastic differential equation (BSDE). This method is particularly...

functionalities and solvers for performing model reduction, such as Galerkin and least-squares Petrov–Galerkin projections. The Pressio ecosystem also offers: (1) pressio4py...

In mathematics and applied mathematics, perturbation theory comprises methods for finding an approximate solution to a problem, by starting from the exact...

two-dimensional case of the general n-dimensional phase space. The phase plane method refers to graphically determining the existence of limit cycles in the solutions...

Cheng, D. T. (2005). "Heritage and early history of the boundary element method". Engineering Analysis with Boundary Elements. 29 (3): 268–302. doi:10.1016/j...