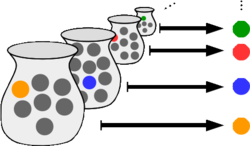
the Vitali convergence theorem, named after the Italian mathematician Giuseppe Vitali, is a generalization of the better-known dominated convergence theorem...

sufficient condition for the convergence of expected values of random variables. Lebesgue's dominated convergence theorem. Let ( f n ) {\displaystyle (f_{n})}...

Various theorems concerning convergence of families of measurable and holomorphic functions, such as Vitali convergence theorem Vitali also proved the existence...

01033. Vitali convergence theorem Vitali covering theorem Vitali–Carathéodory theorem Vitali–Hahn–Saks theorem Vitali set Lebesgue–Vitali theorem J J O'Connor...

This is a generalization of Lebesgue's dominated convergence theorem, see Vitali convergence theorem. Rudin, Walter (1987). Real and Complex Analysis...

function Vitali–Carathéodory theorem, a result in real analysis This disambiguation page lists articles associated with the title Carathéodory's theorem. If...

Schwarz's theorem Interchange of integrals: Fubini's theorem Interchange of limit and integral: Dominated convergence theorem Vitali convergence theorem Fichera...

Vietoris–Begle mapping theorem (algebraic topology) Vinogradov's theorem (number theory) Virial theorem (classical mechanics) Vitali convergence theorem (measure theory)...

In mathematics, the Vitali–Hahn–Saks theorem, introduced by Vitali (1907), Hahn (1922), and Saks (1933), proves that under some conditions a sequence of...

the proof of the Vitali covering theorem. The covering theorem is credited to the Italian mathematician Giuseppe Vitali. The theorem states that it is...

interpolation. The weak (1,1) estimate can be obtained from the Vitali covering lemma. The theorem was first announced by Marcinkiewicz (1939), who showed this...

monotone convergence theorem Fatou's lemma Absolutely continuous Uniform absolute continuity Total variation Radon–Nikodym theorem Fubini's theorem Double...

differential equations. In particular, generalizing the Vitali convergence theorem, the Fichera convergence theorem and previous results of Vladimir Mikhailovich...

differentiation theorem Rademacher differentiation theorem Fatou's theorem on nontangential convergence. Fractional integration theorem Here we use a standard...

Boston. Died: Giuseppe Vitali, 56, Italian mathematician whose name is associated with the Vitali convergence theorem and the Vitali set, from a heart attack...

converge. For example, ∑ 1 ∞ 1 n {\displaystyle \sum _{1}^{\infty }{\frac {1}{n}}} is divergent. dominated Lebesgue's dominated convergence theorem says...

Lebesgue–Vitali theorem Lebesgue spine Lebesgue's lemma Lebesgue's decomposition theorem Lebesgue's density theorem Lebesgue's dominated convergence theorem Lebesgue's...

{\displaystyle a_{n}} satisfying the convergence criterion above is sometimes called a Blaschke sequence. A theorem of Gábor Szegő states that if f ∈ H...

Gaussian measure γ. As stated in the article on the Vitali covering theorem, the Vitali covering theorem fails for Gaussian measures on infinite-dimensional...

Gromov–Hausdorff convergence as an organizing principle.[BGP92] In a followup unpublished paper, Perelman proved his "stability theorem," asserting that...

Lebesgue–Vitali theorem Blaschke–Lebesgue theorem Borel–Lebesgue theorem Fatou–Lebesgue theorem Riemann–Lebesgue lemma Walsh–Lebesgue theorem Dominated...

As an application of the above estimate, we can obtain the Stieltjes–Vitali theorem, which says that that a sequence of holomorphic functions on an open...

the Lebesgue-Vitali theorem (of characterization of the Riemann integrable functions). It has been proven independently by Giuseppe Vitali and by Henri...

"Gromov-Hausdorff convergence" of a sequence of pointed metric spaces to a limit. Gromov formulated an important compactness theorem in this setting, giving...

paper (Jordan 1881). He used the new concept in order to prove a convergence theorem for Fourier series of discontinuous periodic functions whose variation...

mathematician David Milman, who co-authored the Krein–Milman theorem. His brother is the mathematician Vitali Milman. "Milman, Pierre". ISNI. Retrieved 2024-06-12...

B-A\geq 0} It can be seen that a similar result as the Monotone convergence theorem holds for monotone increasing, bounded, self-adjoint operators on...

fundamental theorem of calculus claimed by Harnack. The Cantor function was discussed and popularized by Scheeffer (1884), Lebesgue (1904) and Vitali (1905)...

Euclidean space are Lebesgue measurable; examples of such sets include the Vitali set, and the non-measurable sets postulated by the Hausdorff paradox and...

function there is a Vitali set. The construction of f relies on the axiom of choice. This example can be extended into a general theorem about the existence...