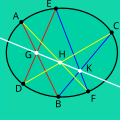
projective geometry, five points determine a conic (a degree-2 plane curve), just as two (distinct) points determine a line (a degree-1 plane curve)...

A conic section, conic or a quadratic curve is a curve obtained from a cone's surface intersecting a plane. The three types of conic section are the hyperbola...

York City Five points determine a conic Five Points Correctional Facility, Romulus, New York Five Points of Calvinism Le Corbusier's Five Points of Architecture...

linear position (no three on a line), there is a pencil of conics through them (five points determine a conic, four points leave one parameter free), of...

that points should impose independent conditions on curves passing through them. For example, five points determine a conic, but in general six points do...

points are allowed to be collinear, and six points are allowed to lie on a conic (in general two points determine a line and five points determine a conic)...

a line (of degree 1) is determined by 2 distinct points on it: one and only one line goes through those two points. Likewise, a non-degenerate conic (polynomial...

to two points determining a line and how five points determine a conic. If two cubics pass through a given set of nine points, then in fact a pencil of...

points on a conic about which special statements can be made, as five points determine a conic. The converse is the Braikenridge–Maclaurin theorem, named for...

coordinates, and five points determine a conic, if the points are in general linear position, as passing through a given point imposes a linear condition...

points determine a conic, but six general points do not lie on a conic, so whether any 6-tuple of points lies on a conic is also a projective invariant...

field any two conics meet in four points (counted with multiplicity) and so, determine the pencil of conics based on these four points. Furthermore, the...

} In geometry, five points determine a conic, so arbitrary sets of six points do not generally lie on a conic section, let alone a circle. Nevertheless...

discovered this result. This hyperbola is the unique conic which passes through the five points A, B, C, G, O. The three triangles △XBC, △YCA, △ZAB erected...

great-circle distances from a pair of foci is a constant Many theorems relating to planar conic sections also extend to spherical conics. If a sphere is intersected...

axioms can construct exactly the same set of points as the extended constructions using a compass and conic drawing tool. Therefore, origami can also be...

zeros, one can consider conics as points in the five-dimensional projective space P 5 . {\displaystyle \mathbf {P} ^{5}.} The conic sections described by...

orbit, 1 is a parabolic escape orbit (or capture orbit), and greater than 1 is a hyperbola. The term derives its name from the parameters of conic sections...

Y\}.} Given a conic C and a point P not on it, two distinct secant lines through P intersect C in four points. These four points determine a quadrangle...

of the five regular three-dimensional regular Platonic solid can have. A conic is determined using five points in the same way that two points are needed...

a c , {\displaystyle b^{2}-ac,} and determines the shape of the conic section. If this discriminant is negative, the curve either has no real points,...

all conics are defined as the solution set of an equation of a plane and of the equation of a cone just given. This formalism allows one to determine the...

parallel, then the surface of revolution is a circular cylinder. In analogy with the conic sections, the set of points whose Cartesian coordinates satisfy the...

Every quadratic equation in X, Y, and Z determines a unique conic, its vanishing locus. Conversely, every conic in the complex projective plane has an...

Bézout's theorem. However, five points are needed to define a nondegenerate conic, so again in this case there is no paradox. In a letter to Euler, Cramer...

diametrically opposite points, called antipodal points. Any two points that are not antipodal points determine a unique great circle. There is a natural unit of...

Steiner's conic problem) and the derivation of the multiple-point formula, the formula allowing one to count or enumerate the points in a fiber even...

determines a curve. He apparently derived these properties of conic sections and others as well. Using this information it was now possible to find a...

response to the problems of navigation, a number of European maritime powers offered prizes for a method to determine longitude at sea. The best-known of...

compass. All points that uniquely define a construction, which can be determined with the use of the compass, are equally determinable without, albeit...