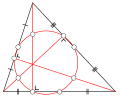
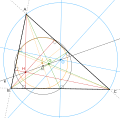
The orthocenter of a triangle, usually denoted by H, is the point where the three (possibly extended) altitudes intersect. The orthocenter lies inside...
19 KB (2,672 words) - 22:01, 22 April 2025
Altitude (triangle) (section Orthocenter)
cosines. The orthocenter of a triangle, usually denoted by H, is the point where the three (possibly extended) altitudes intersect. The orthocenter lies inside...
9 KB (1,371 words) - 05:49, 27 May 2025
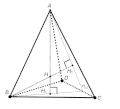
three altitudes intersect in a single point, called the orthocenter of the triangle. The orthocenter lies inside the triangle if and only if the triangle...
55 KB (6,518 words) - 21:25, 19 June 2025
several important points determined from the triangle, including the orthocenter, the circumcenter, the centroid, the Exeter point and the center of the...
18 KB (2,590 words) - 19:02, 22 January 2025
midpoint of the line segment from each vertex of the triangle to the orthocenter (where the three altitudes meet; these line segments lie on their respective...
17 KB (2,124 words) - 08:58, 28 June 2025
orthocentric system is a set of four points on a plane, one of which is the orthocenter of the triangle formed by the other three. Equivalently, the lines passing...
13 KB (1,604 words) - 19:06, 22 January 2025
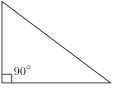
Since these intersect at the right-angled vertex, the right triangle's orthocenter—the intersection of its three altitudes—coincides with the right-angled...
18 KB (2,947 words) - 05:47, 11 May 2025
between that triangle's orthocenter H and circumcenter O. The centroid G also lies on the same line, 2/3 of the way from the orthocenter to the circumcenter...
7 KB (1,088 words) - 08:17, 16 January 2025
orthic axis of △ABC. The isogonal conjugate of the circumcenter X3 is the orthocenter X4 (also denoted by H) having trilinear coordinates sec A : sec B : sec...
13 KB (1,781 words) - 00:54, 15 May 2024
mathematician Gaston Albert Gohierre de Longchamps. It is the reflection of the orthocenter of the triangle about the circumcenter. Let the given triangle have vertices...
6 KB (699 words) - 00:54, 4 February 2024
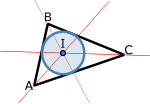
center and points such as a centroid. However, there is generally no orthocenter in the sense of intersecting altitudes. Gaspard Monge found a center...
75 KB (9,506 words) - 16:35, 5 July 2025
circle of the triangle. Together with the centroid, circumcenter, and orthocenter, it is one of the four triangle centers known to the ancient Greeks,...
15 KB (2,225 words) - 00:59, 18 February 2025
Orthocentric tetrahedron (redirect from Orthocenter (tetrahedron))
concurrent. This common point is called the tetrahedron orthocenter (a generalization of the orthocenter of a triangle). It has the property that it is the...
4 KB (498 words) - 21:07, 22 June 2025
while the orthocenter and the circumcenter are in an acute triangle's interior, they are exterior to an obtuse triangle. The orthocenter is the intersection...
13 KB (2,153 words) - 09:46, 10 September 2024
polar circle of a triangle is the circle whose center is the triangle's orthocenter and whose squared radius is r 2 = H A ¯ × H D ¯ = H B ¯ × H E ¯ = H C...
3 KB (329 words) - 09:21, 3 October 2024
of a non-equilateral triangle is the circle that has the triangle's orthocenter and centroid at opposite ends of its diameter. This diameter also contains...
4 KB (403 words) - 09:42, 25 June 2025
states that the orthocenter of the diagonal triangle of a cyclic quadrilateral is the circumcenter of the cyclic quadrilateral. Orthocenter Power of a point...
2 KB (232 words) - 19:15, 26 March 2025
the triangle. For example, the centroid, circumcenter, incenter and orthocenter were familiar to the ancient Greeks, and can be obtained by simple constructions...
32 KB (3,897 words) - 15:45, 3 July 2025
the area of each is 1/4 the area of the original triangle.: p.177 The orthocenter of the medial triangle coincides with the circumcenter of triangle △ABC...
7 KB (878 words) - 17:23, 30 December 2024
geometric theorem that the altitudes of a triangle always meet in a single orthocenter. Gauss was concerned with John Napier's "Pentagramma mirificum" – a certain...
181 KB (17,932 words) - 16:42, 7 July 2025
and orthocenter. The line that passes through all of them is known as the Euler line. The isogonal conjugate of the circumcenter is the orthocenter. The...
27 KB (4,773 words) - 14:15, 18 June 2025
triangle is then △LMN. If △ABC is not an obtuse triangle and P is the orthocenter, then the angles of △LMN are 180° − 2A, 180° − 2B and 180° − 2C. The...
6 KB (849 words) - 11:45, 4 July 2025
orthocenter of the reference triangle, and its nine-point center is known to be that midpoint. Since the central symmetry also maps the orthocenter of...
10 KB (1,255 words) - 20:26, 24 January 2023
distance between the circumcenter and the orthocenter as follows: For the circumcenter pa = aSA and for the orthocenter qa = SBSC/a K p = ∑ cyclic a 2 S A =...
7 KB (1,772 words) - 23:57, 16 May 2025
hyperbola passing through important triangle centers such as the incenter, orthocenter, Gergonne point, Nagel point, mittenpunkt and Schiffler point. The center...
6 KB (712 words) - 21:21, 23 June 2025
excircles has radius 2 R {\displaystyle 2R} . If H {\displaystyle H} is the orthocenter of △ A B C {\displaystyle \triangle ABC} , then r a + r b + r c + r =...
34 KB (5,710 words) - 20:39, 2 April 2025
concerns a property of two perpendicular lines intersecting at a triangle's orthocenter. Harcourt's theorem concerns the relationship of line segments through...
15 KB (2,314 words) - 18:12, 19 May 2025
Neuberg cubic passes through the following points: incenter, circumcenter, orthocenter, both Fermat points, both isodynamic points, the Euler infinity point...
20 KB (2,876 words) - 13:32, 4 July 2025
is continuous: Construct the orthocenter of triangle and three midpoints (say A', B' C' ) between vertices and orthocenter. Construct a circumcircle of...
28 KB (1,770 words) - 16:49, 18 April 2025
incenter, the circumcenter, the nine-point center, the centroid and the orthocenter. In addition to the sides and diagonals of a quadrilateral, some important...
11 KB (1,526 words) - 10:06, 18 May 2025