geometry, Serre's theorem on affineness (also called Serre's cohomological characterization of affineness or Serre's criterion on affineness) is a theorem due...
3 KB (305 words) - 23:40, 5 March 2025
ringed space. A theorem of Serre gives a cohomological characterization of an affine variety; it says an algebraic variety is affine if and only if H...
31 KB (4,377 words) - 22:02, 13 June 2025
enjoyed by all other affine varieties (see Serre's theorem on affineness). But also all of the étale cohomology groups on affine space are trivial. In...
48 KB (7,537 words) - 05:07, 13 April 2025
Coherent sheaf cohomology (redirect from Serre's vanishing theorem)
sheaves on X to coherent analytic sheaves on the associated analytic space Xan. The key GAGA theorem (by Grothendieck, generalizing Serre's theorem on the...
26 KB (4,664 words) - 11:28, 9 October 2024
the mathematical fields of topology and K-theory, the Serre–Swan theorem, also called Swan's theorem, relates the geometric notion of vector bundles to the...
8 KB (1,043 words) - 16:48, 1 February 2024
The Quillen–Suslin theorem, also known as Serre's problem or Serre's conjecture, is a theorem in commutative algebra concerning the relationship between...
6 KB (657 words) - 07:29, 27 December 2024
specific definitions can be shown to be special case of Serre's definition. In the case of Bézout's theorem, the general intersection theory can be avoided,...
24 KB (3,574 words) - 02:08, 16 June 2025
Serre's theorem in group cohomology Serre's theorem on affineness Serre twist sheaf Serre's vanishing theorem Serre weights Thin set in the sense of Serre Serre...
2 KB (140 words) - 18:47, 2 June 2025
Spectrum of a ring (redirect from Affine scheme)
Scheme (mathematics) Projective scheme Spectrum of a matrix Serre's theorem on affineness Étale spectrum Ziegler spectrum Primitive spectrum Stone duality...
25 KB (4,089 words) - 21:05, 8 March 2025
quasi-coherent sheaves F on a noetherian scheme X), then X is Stein (resp. affine); see (Serre 1956) (resp. (Serre 1957) and (Hartshorne 1977, Theorem III.3.7)). Cousin...
4 KB (409 words) - 20:41, 7 March 2024
dimension. This result may be proven using Serre's theorem on regular local rings. Quillen–Suslin theorem Hilbert series and Hilbert polynomial D. Hilbert...
13 KB (2,281 words) - 11:56, 9 June 2025
In mathematics, the Ax–Grothendieck theorem is a result about injectivity and surjectivity of polynomials that was proved independently by James Ax and...
7 KB (920 words) - 20:18, 22 March 2025
Sheaf of algebras (redirect from Affine morphism)
quasi-coherent A {\displaystyle A} -modules. quasi-affine morphism Serre's theorem on affineness EGA 1971, Ch. I, Théorème 9.1.4. EGA 1971, Ch. I, Definition...
5 KB (906 words) - 18:17, 9 July 2025
them (this is discussed at length in Serre's Lectures on the Mordell-Weil theorem). Let A be a thin set in affine n-space over Q and let N(H) denote the...
7 KB (1,021 words) - 22:15, 9 November 2023
Jouanolou's trick (category Mathematical theorems)
Jouanolou's trick is a theorem that asserts, for an algebraic variety X, the existence of a surjection with affine space fibers from an affine variety W to X...
6 KB (1,001 words) - 20:14, 30 January 2025
modules over the ring localized on Serre's subcategory of graded modules of finite length; there is also analogous theorem for coherent sheaves when the...
22 KB (2,408 words) - 07:34, 9 May 2025
of algebraic geometry are fundamental in Wiles's proof of Fermat's Last Theorem, a problem that was stated in terms of elementary arithmetic, and remained...
102 KB (10,065 words) - 16:31, 26 June 2025
particular, Serre's GAGA theorem says that every projective analytic variety is actually an algebraic variety, and the study of holomorphic data on an analytic...
26 KB (3,677 words) - 14:31, 7 September 2023
Glossary of algebraic geometry (redirect from Affine cover)
the dual of Serre's twisting sheaf O X ( 1 ) {\displaystyle {\mathcal {O}}_{X}(1)} . O X ( 1 ) {\displaystyle {\mathcal {O}}_{X}(1)} Serre's twisting sheaf...
82 KB (12,496 words) - 00:02, 12 April 2025
Cohen–Macaulay ring (redirect from Unmixedness theorem)
who proved the unmixedness theorem for polynomial rings, and for Irvin Cohen (1946), who proved the unmixedness theorem for formal power series rings...
23 KB (3,123 words) - 15:42, 27 June 2025
{\displaystyle d=\dim A} , the Krull dimension. See also: Serre's inequality on height and Serre's multiplicity conjectures. Regular local rings were originally...
13 KB (1,922 words) - 20:53, 28 May 2025
Projective variety (redirect from Serre vanishing)
variety is a line bundle of a divisor. Chow's theorem can be shown via Serre's GAGA principle. Its main theorem states: Let X be a projective scheme over...
45 KB (7,499 words) - 13:00, 31 March 2025
Intersection theory (redirect from Serre's Tor formula)
theory for varieties is older, with roots in Bézout's theorem on curves and elimination theory. On the other hand, the topological theory more quickly reached...
17 KB (2,216 words) - 20:50, 8 April 2025
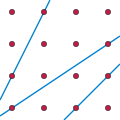
The Sylvester–Gallai theorem in geometry states that every finite set of points in the Euclidean plane has a line that passes through exactly two of the...
41 KB (5,243 words) - 13:09, 24 June 2025
varieties. Serre formulated the Riemann–Roch theorem as a problem of dimension of coherent sheaf cohomology, and also Serre proved Serre duality. Cartan...
124 KB (17,717 words) - 22:01, 1 July 2025
There also is Brauer's theorem on induced characters. In mathematics, Brauer's theorem, named for Richard Brauer, is a result on the representability of...
4 KB (598 words) - 23:11, 31 August 2023
Finite geometry (section Finite affine planes)
order a prime power. The best general result to date is the Bruck–Ryser theorem of 1949, which states: If n is a positive integer of the form 4k + 1 or...
22 KB (2,841 words) - 13:36, 12 April 2024
theorems in Diophantine geometry that are of fundamental importance include: Mordell–Weil theorem Roth's theorem Siegel's theorem Faltings's theorem Serge...
8 KB (935 words) - 19:55, 6 May 2024
Algebraic variety (redirect from Affine curve)
varieties have been called "varieties in the sense of Serre", since Serre's foundational paper FAC on sheaf cohomology was written for them. They remain...
41 KB (5,761 words) - 04:39, 25 May 2025
Carlos Vinuesa, 2010) Serre's modularity conjecture (Chandrashekhar Khare and Jean-Pierre Wintenberger, 2008) Green–Tao theorem (Ben J. Green and Terence...
195 KB (20,072 words) - 22:03, 9 July 2025