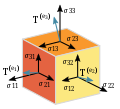
continuum mechanics, the strain-rate tensor or rate-of-strain tensor is a physical quantity that describes the rate of change of the strain (i.e., the relative...

coordinate system, the strain rate tensor can be represented by a symmetric 3×3 matrix of real numbers. The strain rate tensor typically varies with position...

tensor is a tensor used in continuum mechanics to model the part of the stress at a point within some material that can be attributed to the strain rate...

to consider a scalar value for the shear rate by calculating the second invariant of the strain-rate tensor γ ˙ = 2 ε : ε {\displaystyle {\dot {\gamma...

first order approximated by a strain tensor that changes with time. The time derivative of that tensor is the strain rate tensor, that expresses how the element's...

infinitesimal strain tensor ε i j {\displaystyle \varepsilon _{ij}} , similarly to the Cauchy stress tensor, can be expressed as the sum of two other tensors: a...

A strain tensor is defined by the IUPAC as: "A symmetric tensor that results when a deformation gradient tensor is factorized into a rotation tensor followed...

be represented by two-second-order tensors, the strain tensor ε (in lieu of the displacement X) and the stress tensor σ (replacing the restoring force F)...

is determined by tensor invariants of the deviatoric stress tensor τ {\displaystyle {\boldsymbol {\tau }}} and the strain rate tensor ϵ ˙ {\displaystyle...

strain field depending on whether it is defined with respect to the initial or the final configuration of the body and on whether the metric tensor or...

identity tensor), which describes crude shear flow (i.e. the strain rate tensor), pure shear flow (i.e. the deviatoric part of the strain rate tensor, i.e...

through several other rheological properties that relate stress and strain rate tensors under many different flow conditions—such as oscillatory shear or...

Residual stress Shear strength Shot peening Strain Strain tensor Strain rate tensor Stress–energy tensor Stress–strain curve Stress concentration Transient friction...

it is one third of the first invariant of the stress tensor (i.e. the trace of the stress tensor): σ h = I i 3 = 1 3 tr ⁡ ( σ ) {\displaystyle \sigma...

Kolmogorov time scale can be obtained from the inverse of the mean square strain rate tensor, τ η = 1 2 ⟨ E i j E i j ⟩ , {\displaystyle \tau _{\eta }={\tfrac...

designed in the form of a relation between a stress-rate and a strain-rate (or the rate of deformation tensor). The mechanical response of a material should...

the stress variable is the tensor gradient ∇ u {\textstyle \nabla \mathbf {u} } , or more simply the rate-of-strain tensor: ε ( ∇ u ) ≡ 1 2 ∇ u + 1 2...

Enstrophy Group velocity Particle velocity Pressure gradient Strain rate Strain-rate tensor Stream function Velocity potential Vorticity Wind velocity Duderstadt...

three assumptions were made by Stokes: The stress tensor is a linear function of the strain rate tensor or equivalently the velocity gradient. The fluid...

A Rivlin–Ericksen temporal evolution of the strain rate tensor such that the derivative translates and rotates with the flow field. The first-order Rivlin–Ericksen...

directions, eij are the components of the strain rate tensor, Δ is the volumetric strain rate (or dilatation rate) and δij is the Kronecker delta. The ideal...

of the strain rate tensor γ ˙ _ _ {\displaystyle {\underline {\underline {\dot {\gamma }}}}} . Note that the double underlines indicate a tensor quantity...

Electromagnetic tensor (or Faraday tensor) in electromagnetism Finite deformation tensors for describing deformations and strain tensor for strain in continuum...

_{p}} ; D {\displaystyle \mathbf {D} } is the deformation rate tensor or rate of strain tensor, D = 1 2 [ ∇ v + ( ∇ v ) T ] {\displaystyle \mathbf {D} ={\frac...

\mathbf {u} +(\nabla \mathbf {u} )^{T})} be the velocity, pressure and strain rate tensor of the Stokes flow and u ′ ,   p ′ {\displaystyle \mathbf {u} ',\...

Hooke's law) where the Cauchy stress tensor (of order d-1 in d dimensions) is a function of the strain tensor. Although this description is accurate...

or Oldroyd derivative, named after James G. Oldroyd, is the rate of change of some tensor property of a small parcel of fluid that is written in the coordinate...

}}}} is the material rate of the Cauchy stress tensor, and L {\displaystyle {\boldsymbol {L}}} is the spatial velocity gradient tensor. If only these two...

Strain rate imaging is a method in echocardiography (medical ultrasound) for measuring regional or global deformation of the myocardium (heart muscle)...

Malleability Planar deformation features Plasticity (physics) Poisson's ratio Strain tensor Strength of materials Wood warping Rees, David (2006). Basic Engineering...