Inspired by—but distinct from—the Hamiltonian of classical mechanics, the Hamiltonian of optimal control theory was developed by Lev Pontryagin as part...

Molecular Hamiltonian, the Hamiltonian operator representing the energy of the electrons and nuclei in a molecule Hamiltonian (control theory), a function...

it is of fundamental importance in most formulations of quantum theory. The Hamiltonian is named after William Rowan Hamilton, who developed a revolutionary...

Optimal control theory is a branch of control theory that deals with finding a control for a dynamical system over a period of time such that an objective...

equation in control theory Hamilton–Jacobi–Einstein equation In both mathematics and physics (specifically mathematical physics): the term Hamiltonian refers...

The Hamiltonian constraint arises from any theory that admits a Hamiltonian formulation and is reparametrisation-invariant. The Hamiltonian constraint...

In control theory, a bang–bang controller (hysteresis, 2 step or on–off controller), is a feedback controller that switches abruptly between two states...

(generalized) momenta. Both theories provide interpretations of classical mechanics and describe the same physical phenomena. Hamiltonian mechanics has a close...

of time Time translation symmetry Hamiltonian system Propagator Time evolution operator Hamiltonian (control theory) Lecture 1 | Quantum Entanglements...

the Seiberg–Witten gauge theory which reduces SU(2) to U(1) in N = 2, d = 4 gauge theory. The Hamiltonian version of the theory has been developed by Floer...

perturbation theory, the perturbation Hamiltonian is static (i.e., possesses no time dependence). Time-independent perturbation theory was presented...

defined an anomaly-free Hamiltonian operator and showed the existence of a mathematically consistent background-independent theory. The covariant, or "spin...

Stochastic control or stochastic optimal control is a sub field of control theory that deals with the existence of uncertainty either in observations or...

In physics, a gauge theory is a type of field theory in which the Lagrangian, and hence the dynamics of the system itself, do not change under local transformations...

milestone in optimal control theory, the significance of the maximum principle lies in the fact that maximizing the Hamiltonian is much easier than the...

Møller–Plesset perturbation theory uses the difference between the Hartree–Fock Hamiltonian and the exact non-relativistic Hamiltonian as the perturbation. The...

time-independent Hamiltonian HS, where H0,S is the free Hamiltonian, Duck, Ian; Sudarshan, E.C.G. (1998). "Chapter 6: Dirac's Invention of Quantum Field Theory". Pauli...

optimal control by taking the maximizer (or minimizer) of the Hamiltonian involved in the HJB equation. The equation is a result of the theory of dynamic...

Probability theory or probability calculus is the branch of mathematics concerned with probability. Although there are several different probability interpretations...

and corresponding generalized velocities in configuration space) and Hamiltonian mechanics (using coordinates and corresponding momenta in phase space)...

that includes every vertex of the graph without repeats is known as a Hamiltonian path. Two paths are vertex-independent (alternatively, internally disjoint...

field theory Classical unified field theories Variational methods in general relativity Higgs field (classical) Lagrangian (field theory) Hamiltonian field...

Chaos theory is an interdisciplinary area of scientific study and branch of mathematics. It focuses on underlying patterns and deterministic laws of dynamical...

}}e^{-ip_{\mu }(x^{\mu }-y^{\mu })}.} In an interacting theory, where the Lagrangian or Hamiltonian contains terms L I ( t ) {\displaystyle L_{I}(t)} or...

(generalized) momenta. Both theories provide interpretations of classical mechanics and describe the same physical phenomena. Hamiltonian mechanics has a close...

Coding theory is the study of the properties of codes and their respective fitness for specific applications. Codes are used for data compression, cryptography...

In physics, string theory is a theoretical framework in which the point-like particles of particle physics are replaced by one-dimensional objects called...

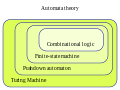
Automata theory is the study of abstract machines and automata, as well as the computational problems that can be solved using them. It is a theory in theoretical...

A conformal field theory (CFT) is a quantum field theory that is invariant under conformal transformations. In two dimensions, there is an infinite-dimensional...

physics of the system will be described by a certain formula, say the Hamiltonian H(T, J). Now proceed to divide the solid into blocks of 2×2 squares;...