在數學分析 中,半連續性 是實值函數 的一種性質,分成上半連續 與下半連續 ,半連續性較連續性 弱。
設 X {\displaystyle X} 拓撲空間 , x 0 ∈ X {\displaystyle x_{0}\in X} f : X → R {\displaystyle f:X\to \mathbb {R} } 函數 。若對每個 ε > 0 都存在 x 0 {\displaystyle x_{0}} U {\displaystyle U} ∀ x ∈ U , f ( x ) < f ( x 0 ) + ε {\displaystyle \forall x\in U,\;f(x)<f(x_{0})+\varepsilon } f {\displaystyle f} x 0 {\displaystyle x_{0}} 上半連續 。該條件也可以用上極限 等價地表述:
lim sup x → x 0 f ( x ) ≤ f ( x 0 ) {\displaystyle \limsup _{x\to x_{0}}f(x)\leq f(x_{0})} 若 f {\displaystyle f} X {\displaystyle X} 上半連續函數 。
下半連續性可以準此定義:若對每個 ε > 0 都存在 x 0 {\displaystyle x_{0}} U {\displaystyle U} ∀ x ∈ U , f ( x ) > f ( x 0 ) − ε {\displaystyle \forall x\in U,\;f(x)>f(x_{0})-\varepsilon } f {\displaystyle f} x 0 {\displaystyle x_{0}} 下半連續 。用下極限 等價地表述為:
lim inf x → x 0 f ( x ) ≥ f ( x 0 ) {\displaystyle \liminf _{x\to x_{0}}f(x)\geq f(x_{0})} 若 f {\displaystyle f} X {\displaystyle X} 下半連續函數 。
拓撲基 ] − ∞ , a [ ( a ∈ R ) {\displaystyle ]-\infty ,a[\;\;(a\in \mathbb {R} )} R {\displaystyle \mathbb {R} } ] a , + ∞ [ ( a ∈ R ) {\displaystyle ]a,+\infty [\;\;(a\in \mathbb {R} )}
上半連續但不是下半連續函數的例子(藍點表 f ( x 0 ) {\displaystyle f(x_{0})} 考慮函數
f ( x ) = { − 1 , x < 0 1 , x ≥ 0 {\displaystyle f(x)={\begin{cases}-1&,x<0\\1&,x\geq 0\end{cases}}} 此函數在 x 0 = 0 {\displaystyle x_{0}=0}
下半連續但不是上半連續连续的函数的例子(藍點表 f ( x 0 ) {\displaystyle f(x_{0})} 下整數函數 f ( x ) = ⌊ x ⌋ {\displaystyle f(x)=\lfloor x\rfloor } f ( x ) = ⌈ x ⌉ {\displaystyle f(x)=\lceil x\rceil }
一個函數在一點連續的充要條件是它在該點既上半連續也下半連續。
若 f , g {\displaystyle f,g} f + g {\displaystyle f+g} f g {\displaystyle fg} f {\displaystyle f} − f {\displaystyle -f}
若 X {\displaystyle X}
設 f n {\displaystyle f_{n}} x ∈ X {\displaystyle x\in X}
f ( x ) = sup n f n ( x ) < + ∞ {\displaystyle f(x)=\sup _{n}f_{n}(x)<+\infty } 則 f {\displaystyle f}
開集的指示函數 為下半連續函數,閉集的指示函數為上半連續函數。
Hyers, Donald H.; Isac, George; Rassias, Themistocles M. Topics in nonlinear analysis & applications. World Scientific. 1997. ISBN 9810225342 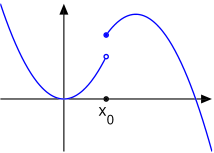



 French
French Deutsch
Deutsch









![{\displaystyle ]-\infty ,a[\;\;(a\in \mathbb {R} )}](https://wikimedia.org/api/rest_v1/media/math/render/svg/98ff6c9fb09f84672c03acfb7f8fb6a7b607abf2)

![{\displaystyle ]a,+\infty [\;\;(a\in \mathbb {R} )}](https://wikimedia.org/api/rest_v1/media/math/render/svg/93a749d114179cc28d559203f6d9f33f1c12d673)











