多项式 x 2 + c x + d {\displaystyle x^{2}+cx+d} ( x + a ) ( x + b ) {\displaystyle \left(x+a\right)\left(x+b\right)} a b = d , a + b = c {\displaystyle ab=d,a+b=c} 因式分解 ,在这里是指多項式因式分解 (英語:Polynomial Factorization [ 註 1] 多項式 分解為兩個或多個的因式[ 註 2] x 2 − 1 2 {\displaystyle x^{2}-1^{2}} ( x + 1 ) ( x − 1 ) {\displaystyle \left(x+1\right)\left(x-1\right)} x 2 − y 2 {\displaystyle x^{2}-y^{2}} ( x + y ) ( x − y ) {\displaystyle \left(x+y\right)\left(x-y\right)} 複整數 ,那么 x 2 + 1 2 {\displaystyle x^{2}+1^{2}} ( x + i ) ( x − i ) {\displaystyle \left(x+i\right)\left(x-i\right)} 不可约多项式 (irreducible )。也就是不能再分解了。
数域 P {\displaystyle P} f ( x ) {\displaystyle f(x)} p i ( x ) {\displaystyle p_{i}(x)}
在复数域上,每个不可约多项式都是一次的,因此高于一次的复系数多项式,都可以唯一地分解为多个一次式之积。
在实数域上,不可约的多项式都是一次或二次的,因此高于一次的实系数多项式,都可以唯一地分解为一次、二次多项式之积。
在有理数域上,不可约多项式可以有任何次。例如,在有理数范围内,当 n {\displaystyle n} x {\displaystyle x} x n + 2 {\displaystyle x^{n}+2} [ 1]
数域F上每个次数 ≥ 1 {\displaystyle \geq 1} f ( x ) {\displaystyle f(x)}
f ( x ) = p 1 ( x ) p 2 ( x ) p 3 ( x ) ⋯ p s ( x ) = q 1 ( x ) q 2 ( x ) ⋯ q t ( x ) {\displaystyle f(x)=p_{1}(x)p_{2}(x)p_{3}(x)\cdots p_{s}(x)=q_{1}(x)q_{2}(x)\cdots q_{t}(x)}
其中 p i ( x ) ( i = 1 , 2 , ⋯ , s ) {\displaystyle p_{i}(x)(i=1,2,\cdots ,s)} q j ( x ) ( j = 1 , 2 , ⋯ , t ) {\displaystyle q_{j}(x)(j=1,2,\cdots ,t)} s = t {\displaystyle s=t}
p i ( x ) = c i q i ( x ) ( i = 1 , 2 , ⋯ , s ) {\displaystyle p_{i}(x)=c_{i}q_{i}(x)(i=1,2,\cdots ,s)} c i ( i = 1 , 2 , ⋯ , s ) {\displaystyle c_{i}(i=1,2,\cdots ,s)}
原则:
分解必須 要彻底(即分解後之因式均不能再做分解) 結果最後只留下小括號 結果的多項式首項為正。 在一個公式內把其公因子抽出,例子:
7 a + 98 a b {\displaystyle 7a+98ab} 其中, 7 a {\displaystyle 7a} 7 a ( 1 + 14 b ) {\displaystyle 7a(1+14b)} 51 a 4 b 7 + 24 a 3 b 2 + 75 a 5 b 5 {\displaystyle 51a^{4}b^{7}+24a^{3}b^{2}+75a^{5}b^{5}} 其中, 3 a 3 b 2 {\displaystyle 3a^{3}b^{2}} 3 a 3 b 2 ( 17 a b 5 + 25 a 2 b 3 + 8 ) {\displaystyle 3a^{3}b^{2}(17ab^{5}+25a^{2}b^{3}+8)} 兩個立方數之和 a 3 + b 3 = ( a + b ) ( a 2 − a b + b 2 ) {\displaystyle a^{3}+b^{3}=(a+b)(a^{2}-ab+b^{2})}
兩個立方數之差 a 3 − b 3 = ( a − b ) ( a 2 + a b + b 2 ) {\displaystyle a^{3}-b^{3}=(a-b)(a^{2}+ab+b^{2})}
兩個n次方數之差 a n − b n = ( a − b ) ( a n − 1 + a n − 2 b + . . . . . . + b n − 1 ) {\displaystyle a^{n}-b^{n}=(a-b)(a^{n-1}+a^{n-2}b+......+b^{n-1})}
兩個奇數次方數之和 a n + b n = ( a + b ) ( a n − 1 − a n − 2 b + . . . . . . + b n − 1 ) {\displaystyle a^{n}+b^{n}=(a+b)(a^{n-1}-a^{n-2}b+......+b^{n-1})}
透過公式重組,然後再抽出公因數,例子:
3 a 2 b − 5 y + 12 a 3 b 2 − 20 a b y = ( 3 a 2 b + 12 a 3 b 2 ) − ( 5 y + 20 a b y ) = 3 a 2 b ( 1 + 4 a b ) − 5 y ( 1 + 4 a b ) = ( 1 + 4 a b ) ( 3 a 2 b − 5 y ) {\displaystyle {\begin{aligned}&3a^{2}b-5y+12a^{3}b^{2}-20aby\\=&(3a^{2}b+12a^{3}b^{2})-(5y+20aby)\\=&3a^{2}b(1+4ab)-5y(1+4ab)\\=&(1+4ab)(3a^{2}b-5y)\end{aligned}}}
15 n 2 + 2 m − 3 n − 10 m n = ( 15 n 2 − 3 n ) + ( 2 m − 10 m n ) = 3 n ( 5 n − 1 ) + 2 m ( 1 − 5 n ) = 3 n ( 5 n − 1 ) − 2 m ( 5 n − 1 ) = ( 5 n − 1 ) ( 3 n − 2 m ) {\displaystyle {\begin{aligned}&15n^{2}+2m-3n-10mn\\=&(15n^{2}-3n)+(2m-10mn)\\=&3n(5n-1)+2m(1-5n)\\=&3n(5n-1)-2m(5n-1)=&(5n-1)(3n-2m)\end{aligned}}}
透過添項然後減掉,然後再抽出公因數,例子:
x 4 + x 2 + 1 = x 4 + x 2 + x 2 − x 2 + 1 = x 4 + 2 x 2 − x 2 + 1 = x 4 + 2 x 2 + 1 − x 2 = ( x 2 + 1 ) 2 − x 2 = ( x 2 + 1 − x ) ( x 2 + 1 + x ) = ( x 2 − x + 1 ) ( x 2 + x + 1 ) {\displaystyle {\begin{aligned}&x^{4}+x^{2}+1\\=&x^{4}+x^{2}+x^{2}-x^{2}+1\\=&x^{4}+2x^{2}-x^{2}+1\\=&x^{4}+2x^{2}+1-x^{2}\\=&\left(x^{2}+1\right)^{2}-x^{2}\\=&\left(x^{2}+1-x\right)\left(x^{2}+1+x\right)\\=&\left(x^{2}-x+1\right)\left(x^{2}+x+1\right)\end{aligned}}}
或者透過分裂某項,然後再抽出公因數,例子:
x 3 − 7 x + 6 {\displaystyle x^{3}-7x+6}
其中, − 7 x {\displaystyle -7x} − x {\displaystyle -x} − 6 x {\displaystyle -6x} x 3 − 7 x + 6 {\displaystyle x^{3}-7x+6} x 3 − x − 6 x + 6 {\displaystyle x^{3}-x-6x+6}
x 3 − 7 x + 6 = x 3 − x − 6 x + 6 = ( x 3 − x ) − ( 6 x − 6 ) = x ( x 2 − 1 ) − 6 ( x − 1 ) = x ( x + 1 ) ( x − 1 ) − 6 ( x − 1 ) = [ x ( x + 1 ) − 6 ] ( x − 1 ) = ( x 2 + x − 6 ) ( x − 1 ) {\displaystyle {\begin{aligned}&x^{3}-7x+6\\=&x^{3}-x-6x+6\\=&\left(x^{3}-x\right)-\left(6x-6\right)\\=&x\left(x^{2}-1\right)-6\left(x-1\right)\\=&x\left(x+1\right)\left(x-1\right)-6\left(x-1\right)\\=&\left[x\left(x+1\right)-6\right]\left(x-1\right)\\=&\left(x^{2}+x-6\right)\left(x-1\right)\end{aligned}}}
其中, + x {\displaystyle +x} + 3 x {\displaystyle +3x} − 2 x {\displaystyle -2x} x 2 + x − 6 {\displaystyle x^{2}+x-6} x 2 + 3 x − 2 x − 6 {\displaystyle x^{2}+3x-2x-6}
( x 2 + x − 6 ) ( x − 1 ) = ( x 2 + 3 x − 2 x − 6 ) ( x − 1 ) = [ ( x 2 + 3 x ) − ( 2 x + 6 ) ] ( x − 1 ) = ( x ( x + 3 ) − 2 ( x + 3 ) ) ( x − 1 ) = ( x − 2 ) ( x + 3 ) ( x − 1 ) = ( x − 1 ) ( x − 2 ) ( x + 3 ) {\displaystyle {\begin{aligned}&\left(x^{2}+x-6\right)\left(x-1\right)\\=&\left(x^{2}+3x-2x-6\right)\left(x-1\right)\\=&\left[\left(x^{2}+3x\right)-\left(2x+6\right)\right]\left(x-1\right)\\=&\left(x\left(x+3\right)-2\left(x+3\right)\right)\left(x-1\right)\\=&\left(x-2\right)\left(x+3\right)\left(x-1\right)\\=&\left(x-1\right)\left(x-2\right)\left(x+3\right)\end{aligned}}}
十字交乘法(cross method),也叫做十字相乘法。它实際上是拆項法 的一個變形,只不過用十字形矩陣來表示。
一個整係數的一元多項式 a n x n + a n − 1 x n − 1 + . . . . . . a 1 x + a 0 {\displaystyle a_{n}x^{n}+a_{n-1}x^{n-1}+......a_{1}x+a_{0}} p x + q {\displaystyle px+q} 且p,q互質 ,則以下兩條必成立:(逆敘述並不真)
p | a n {\displaystyle p|a_{n}} q | a 0 {\displaystyle q|a_{0}} 不過反過來說,即使當 p | a n {\displaystyle p|a_{n}} q | a 0 {\displaystyle q|a_{0}} p x + q {\displaystyle px+q} a n x n + a n − 1 x n − 1 + . . . . . . a 1 x + a 0 {\displaystyle a_{n}x^{n}+a_{n-1}x^{n-1}+......a_{1}x+a_{0}}
另外一個看法是:
一個整係數的n次多項式 a n x n + a n − 1 x n − 1 + . . . . . . a 1 x + a 0 {\displaystyle a_{n}x^{n}+a_{n-1}x^{n-1}+......a_{1}x+a_{0}} p x − q {\displaystyle px-q} 且p,q互質 ,則:(逆敘述並不真)
p − q | f ( 1 ) {\displaystyle p-q|f(1)} p + q | f ( − 1 ) {\displaystyle p+q|f(-1)} Burnside, William Snow; Panton, Arthur William (1960) [1912], The Theory of Equations with an introduction to the theory of binary algebraic forms (Volume one), Dover Dickson, Leonard Eugene (1922), First Course in the Theory of Equations, New York: John Wiley & Sons Fite, William Benjamin (1921), College Algebra (Revised), Boston: D. C. Heath & Co. Klein, Felix (1925), Elementary Mathematics from an Advanced Standpoint; Arithmetic, Algebra, Analysis, Dover Selby, Samuel M., CRC Standard Mathematical Tables (18th ed.), The Chemical Rubber Co ^ 多 项 式 (PDF) . 清华大学出版社. [2025-01-04 ] . 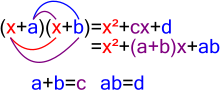


 French
French Deutsch
Deutsch







































![{\displaystyle {\begin{aligned}&x^{3}-7x+6\\=&x^{3}-x-6x+6\\=&\left(x^{3}-x\right)-\left(6x-6\right)\\=&x\left(x^{2}-1\right)-6\left(x-1\right)\\=&x\left(x+1\right)\left(x-1\right)-6\left(x-1\right)\\=&\left[x\left(x+1\right)-6\right]\left(x-1\right)\\=&\left(x^{2}+x-6\right)\left(x-1\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e173081941a261a4a517cd7652052ef66b5d5f4f)





![{\displaystyle {\begin{aligned}&\left(x^{2}+x-6\right)\left(x-1\right)\\=&\left(x^{2}+3x-2x-6\right)\left(x-1\right)\\=&\left[\left(x^{2}+3x\right)-\left(2x+6\right)\right]\left(x-1\right)\\=&\left(x\left(x+3\right)-2\left(x+3\right)\right)\left(x-1\right)\\=&\left(x-2\right)\left(x+3\right)\left(x-1\right)\\=&\left(x-1\right)\left(x-2\right)\left(x+3\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/eb483e17739ac90192cc6ff1f789567260990a3f)






